SAT and SAT Subject Test Dates

500 New SAT Math Problems – Just 19.99 Today Only

June 30, 2024

$500 New SAT Math Problems$

500 New SAT Math Problems
Just 19.99 on Amazon

Hi everyone! The latest edition of 500 New SAT Math Problems is now available in paperback from Amazon. This edition just has been modified from the previous edition to account for the changes on the Digital SAT.

~~The paperback is now on sale on Amazon for only $19.99. Note that once the sale ends (by the end of today), the price of this book will go up to $42.99.~~

The promotion has ended. Thanks to everyone who participated. The book is now available at its regular price here: 500 New SAT Math Problems

If you have any questions, feel free to contact me at steve@SATPrepGet800.com

Thank you all for your continued support!

A Trick For Free Two Day Shipping

I would like to finish this post with a little trick you can use to get free 2 day shipping on any of the books you decide to purchase without making any additional purchases. If you have never used Amazon Prime you can sign up for a free month using the following link.

If you have already had a free trial of Amazon Prime you can simply open up a new Amazon account to get a new free trial. It just takes a few minutes! You will need to use a different email address than the one you usually use.

This next part is very important! After you finish your transaction, go to your Account, select “Manage my prime membership,” and turn off the recurring billing. This way in a month’s time Amazon will not start charging you for the service.

After shutting off the recurring billing you will still continue to receive the benefit of free 2 day shipping for one month. This means that as long as you use this new Amazon account for your purchases you can do all of your shopping on Amazon for the next month without having to worry about placing minimum orders to get free shipping.

Just be aware that certain products from outside sellers do not always qualify for free shipping, so please always check over your bill carefully before you check out.

Well I hope you decide to take advantage of this very special offer, or at the very least I hope you will benefit from my Amazon “free 2 day shipping trick.” Here is the link one more time:

If you think your friends might be interested in this special offer, please share it with them on Facebook:

Thank you all for your continued support!

SAT and SAT Subject Test Dates

August 26, 2016

SAT and SAT Subject Test Dates

The following link will take you to the College Board’s web page showing you the upcoming dates for both the SAT Reasoning Test and the SAT Subject Tests. You can then register right from that page:

Test Dates and Deadlines

If you are an international student, then you should register using the following page instead:

International Registration

And if you are ready to begin preparing for the SAT, you may want to take a look at the Get 800 collection of SAT prep books. Click the follwing picture to see what we offer:
If you think your friends might be interested in this special offer, please share it with them on Facebook:

Increasing Your SAT Math Score from 750 to 800

August 22, 2016

Increasing Your SAT Math Score from 750 to 800

Recently I’ve been getting emails from students that are already scoring over 700 in math on SAT practice tests, but they are still struggling to get a perfect score. First, I’d like to point out that if you are already close to the mid 700’s, then there aren’t any universities that will exclude you based on your SAT math score. On the other hand, I do realize that there are many students out there that would still love to get a perfect score anyway.

The video above shows me completing an SAT math section perfectly in about one-third the time given. Even though this video was for the old SAT, if you are above a 700 in SAT math, it’s worth watching so you can compare what I’m doing to what you’re doing. I also have videos for the other two sections at the bottom of this post.

If you’re one of those students that is struggling to get those last few hard questions right, you may want to take a look at the following article: Stop Getting Those Last Few Hard SAT Math Questions Wrong

If you’re still making careless errors on some of the easier problems, then take a look here: Stop Making Careless Errors in SAT Math

Another possibility is that you’re running out of time, and you don’t have time to catch a careless error or two that you’re still making. Here are some long-term strategies for getting you to increase your speed without feeling rushed.

Don’t just try to solve each problem, figure out how many ways you can solve each problem – using multiple methods multiplies the value you get out of each problem.
After trying to solve each problem several different ways, ask yourself questions about each method: Which one was quickest for you? Which one should have been the quickest, if only you were better at it? Which one guarantees the right answer? Which one was tricky, but more effective once you got the hang of it.
Internalize strategies and methods – don’t just practice them, try to get to a point where they become a part of you.

The goal is to decrease the amount of time spent on each problem by knowing which method to use right away, and having practiced the method enough so that you can arrive at an answer fairly quickly. Never try to decrease the amount of time by physically speeding up! The speed will come with practice, internalizing the strategies, and gaining enough intuition to choose the best method for each individual problem.

The journey from a 750 to an 800 requires some time, some effort, and a lot of practice. Understanding strategies, concepts and methods is no longer enough – you have to make them a part of you so that the ideas form before you even have a chance to think about them. This requires a jump in your level of mathematical maturity. The good news is that if you put in the effort to do this now, then that experience will stay with you, and it will even make your college classes much easier.

SAT Math Practice Problems

For daily SAT math practice, check out the Get 800 collection of SAT math books. Click on the picture below for more information about these books.

And if you liked this article, please share it with your Facebook friends:

Hard ACT Math Probability Problem with Solution

August 20, 2016

Hard ACT Math Probability Problem with Solution

Today I will give a solution to yesterday’s ACT math probability problem. Here is the question again followed by a ful solution.

Level 5 Probability

Suppose that x will be randomly selected from the set

{–3/2, –1, –1/2, 0, 5/2}

and that y will be randomly selected from the set

{–3/4, –1/4, 2, 11/4}.

What is the probability that x/y < 0 ?

A. 1/100
B. 1/20
C. 3/20
D. 1/3
E. 2/5

Solution: There are 5 possible values for x and 4 possible values for y. By the counting principle, there are 5 ⋅ 4 = 20 possibilities for xy (possibly with some repetition).

In order for x/y < 0 to be true, x and y need to be opposite in sign. The number of ways for this to happen (possibly with repetition) is

3 ⋅ 2 + 1 ⋅ 2 = 6 + 2 = 8

So the desired probability is 8/20 = 2/5, choice E.

Notes: (1) In this question when we mention the possibilities for xy, we are ignoring the fact that there could be repeated values. For example, we have (0)(–3/4) = 0 and (0)(–1/4) = 0. Technically these two computations give a single value for xy. Nonetheless, to compute the desired probability we need to think of these as distinct possibilities.

(2) There are 3 negative values for x and 2 positive values for y. This gives us 3 ⋅ 2 = 6 negative values for xy.

There is 1 positive value for x and 2 negative values for y. This gives us 1 ⋅ 2 = 2 more negative values for xy.

So altogether there are 6 + 2 = 8 possibilities that lead to a negative value for xy.

Hard ACT Math Probability Problem

Today I would like to give a hard ACT math probability problem. I will provide a full explanation for this problem tomorrow. In the meantime, feel free to leave your own solutions in the comments.

Level 5 Probability

Suppose that x will be randomly selected from the set

{–3/2, –1, –1/2, 0, 5/2}

and that y will be randomly selected from the set

{–3/4, –1/4, 2, 11/4}.

What is the probability that x/y < 0 ?

A. 1/100
B. 1/20
C. 3/20
D. 1/3
E. 2/5

ACT Math Number Theory Problem with Solutions

Today I will give a solution to yesterday’s ACT math number theory problem. Here is the question one more time followed by a full solution.

Level 3 Number Theory

What positive number when divided by its reciprocal has a result of 9/16?

A. 8/3
B. 3/8
C. 4/3
D. 3/16
E. 3/4

Solution by starting with choice C: Let’s start with 4/3 as our first guess. The reciprocal of 4/3 is 3/4, and when we divide 4/3 by 3/4, we get 16/9. This is not correct, but it is the reciprocal of what we are trying to get. So the answer is the reciprocal of 4/3, which is 3/4, choice E.

Notes: (1) The reciprocal of the fraction a/b is the fraction b/a. In other words, we get the reciprocal of the fraction by interchanging the number on top (the numerator) with the number on bottom (the denominator).

(2) We can divide 4/3 by 3/4 right in our TI-84 calculator by typing

(4 / 3) / (3 / 4) ENTER MATH ENTER ENTER

The output will be 16/9.

Pressing MATH ENTER ENTER at the end changes the decimal to a fraction.

(3) We can also do the computation by hand as follows:

$\frac{4}{3}\div\frac{3}{4}= \frac{4}{3}\cdot\frac{4}{3}= \frac{4\cdot 4}{3\cdot 3}=\frac{16}{9}$

(4) Let’s also just confirm that choice E is the answer. I’ll use the hand method, but you can also feel free to use your calculator.

$\frac{3}{4}\div\frac{4}{3}= \frac{3}{4}\cdot\frac{3}{4}= \frac{3\cdot 3}{4\cdot 4}=\frac{9}{16}$

* Algebraic solution: Let x be the positive number. We are given that

$x\div \frac{1}{x}=\frac{9}{16}$

$\frac{x\cdot x}{1}=\frac{9}{16}$

$x\cdot x=\frac{9}{16}$

$x^2=\frac{9}{16}$

$x=\pm \sqrt{\frac{9}{16}}=\pm \frac{\sqrt{9}}{\sqrt{16}}=\pm \frac{3}{4}$

Since we are given that x is a positive number, x = 3/4, choice E.

ACT Math Number Theory Problem

Today I would like to give an ACT math number theory problem. I will provide a full explanation for this problem tomorrow. In the meantime, feel free to leave your own solutions in the comments.

Level 3 Number Theory

What positive number when divided by its reciprocal has a result of 9/16?

A. 8/3
B. 3/8
C. 4/3
D. 3/16
E. 3/4

Breakdown of the SAT Math Sections

Today I would like to tell you exactly what types of math problems to expect on the SAT. This information comes right from the College Board. The information I give here will probably be enough for most of you, but if you want more information right from the College Board, you can download the full document here: About the SAT Math Test

There are two math sections on the SAT: one where a calculator is allowed, and one where a calculator is not allowed. The following is a breakdown of the exact number of questions of each type that you can expect in each of these sections.

“Calculator Allowed” Section

Heart of Algebra: 11 questions, 29% of section
Geometry, Trigonometry, and Complex Numbers: 3 questions, 8% of section
Passport to Advanced Math: 7 questions, 18% of section
Problem Solving and Data Analysis: 17 questions, 45% of section
Total: 38 questions

“No Calculator” Section

Heart of Algebra: 8 questions, 40% of section
Geometry, Trigonometry, and Complex Numbers: 3 questions, 15% of section
Passport to Advanced Math: 9 questions, 45% of section
Problem Solving and Data Analysis: 0 questions, 0% of section
Total: 20 questions

If you are preparing for the SAT, you may want to check out this special deal on all 8 of my SAT math prep books:

Get Eight Books for the Price of Three

If you think your friends might be interested in the information presented here, please share this post with them on Facebook:

Thank you all for your continued support!

AP Calculus BC Problem with Solution – Arc Length

August 10, 2016

AP Calculus BC Problem with Solution – Arc Length

Today I will give a solution to the AP Calculus BC Arc Length problem I gave yesterday.

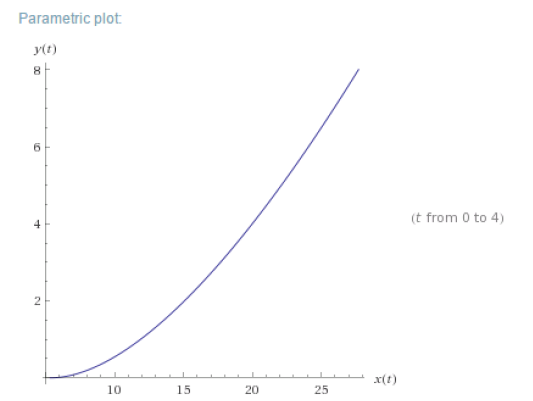
Level 2 Arc Length

Find the length of the arc of the curve defined by

$x(t)=\frac{1}{12}(8t+16)^{\frac{3}{2}}, \quad y(t)=\frac{t^2}{2}$

from t = 0 to t = 4.

Solution:

$\frac{dx}{dt}=(\frac{1}{12})(\frac{3}{2})(8t+16)^{\frac{1}{2}}(8)=\sqrt{8t+16}$

and

$\frac{dy}{dt}=t$

So,

$(\frac{dx}{dt})^2=8t+16$

and

$(\frac{dy}{dt})^2=t^2$

So the desired length is

$\int_0^4 \sqrt{8t+16+t^2} \ dt$

$=\int_0^4 \sqrt{t^2+8t+16} \ dt$

$=\int_0^4 \sqrt{(t+4)^2} \ dt$

$=\int_0^4 (t+4) \ dt$

$=(\frac{t^2}{2}+4t)|_0^4$

$=(\frac{4^2}{2}+4\cdot 4)$

$=8+16$

$=\bf{24}$

Notes: (1) The arc length of the differentiable curve with parametric equations x = x(t) and y = y(t) from a to b is

$\text{Arc Length}=\int_a^b \sqrt{(\frac{dx}{dt})^2+(\frac{dy}{dt})^2} \ dt$

(2) We used the chain rule to compute dx/dt and a simple power rule to compute dy/dt. See problem 2 in 320 AP Calculus BC Problems for more information on the chain rule.

AP Calculus BC Problem- Arc Length

Today I would like to give an AP Calculus BC problem involving arc length. I will provide a full explanation for this problem tomorrow. In the meantime, feel free to leave your own solutions in the comments.

Level 2 Arc Length

Find the length of the arc of the curve defined by

x(t) = 1/12 (8t + 16)^3/2 and y(t) = t²/2,

from t = 0 to t = 4.

The Differences Between the PSAT and the SAT

If you have been reading this blog, then you are probably familiar with what the SAT is. In case you are not, here is a link to one of my articles that gives detailed information on this: Overview of the Math Sections of the SAT

Today we will discuss the PSAT and how it is different from the SAT.

First, did you know that the “P” in PSAT stands for preliminary? Most people incorrectly think that it stands for practice.

Also, almost twice the number of test takers take the PSAT compared to the SAT. This is because the PSAT determines eligibility and qualification for the National Merit Scholarship Program.

Note that unless you get a score high enough to qualify for National Merit (which is a very high score), the PSAT is essentially meaningless. The PSAT is not used for college admissions. It is however a decent way to get an idea of how well you would perform on the SAT.

In addition to College Board Blue Book tests, almost all of my students take the PSAT to gauge how well they are going to do on the SAT.

There are a few major differences between the PSAT and the SAT and this post will shed some light on these differences.

Test Length and Structure

The PSAT consists of 4 sections. There is a 60 minute reading section with 47 questions, a 35 minute writing and language section with 44 questions, a 25 minute math section with 17 questions where a calculator is not allowed, and a 45 minute math section with 31 questions where a calculator is allowed. This gives a total testing time of 60 + 35 + 25 + 45 = 160 minutes, or 2 hours and 40 minutes.

The SAT is slightly longer than the PSAT. It also consists of 4 sections. There is a 65 minute reading section with 52 questions, a 35 minute writing and language section with 44 questions, a 25 minute math section with 20 questions where a calculator is not allowed, and a 55 minute math section with 38 questions where a calculator is allowed. This gives a total required testing time of 65 + 35 + 25 + 55 = 180 minutes, or 3 hours. The SAT is the first, and maybe only test that you will ever take that requires this kind of time commitment.

Scoring

The PSAT is graded out of 1520 points with reading and writing scored between 160 and 760, and math also scored between 160 and 760.

The SAT is graded out of 1600 points with reading and writing consisting of a total of 800 points, and math also consisting of a total of 800 points.

Note that the two scales are similar, but the maximum possible PSAT score is lower than the maximum possible SAT score. This is due to the fact that the PSAT is a bit easier than the SAT.

You can essentially use your PSAT score as an estimate to what you might get on the SAT. Although the highest possible score on the PSAT is 1520 as compared to 1600 on the SAT, due to the fact that the SAT is more difficult than the PSAT, your SAT score would not be higher than your PSAT score. It would probably be approximately the same.

Other than this small score discrepancy all other aspects of scoring the PSAT and the SAT are the same. Multiple choice questions and grid ins are handled the same way, and the raw score is computed in the same fashion. For more details on how this is done, see this article titled Overview of the Math Sections of the SAT.

Just one comment on the scaled score – although for most students the scaled score is handled almost identically on both the PSAT and SAT, the strongest students may be penalized a bit more heavily on the PSAT. For example just one wrong question on the math section of the PSAT could drop your scaled score down 50 points. This could really hurt if you are applying for a National Merit Scholarship.

Final Note

As mentioned in the section on scoring above, if you decide to take your SAT without any preparation, then it is likely that your score will not go up from your PSAT score. So whether you use a tutor, take a prep course, or prepare on your own, please prepare. With good preparation you can show a significant increase in score from your PSAT to your SAT.

If you do decide to study on your own, I suggest you take a look at my “28 SAT Math Lessons” series. Each book in this series is targeted for students in a specific score range. Get the book for your score range and you will learn all the strategies you need to get to the next score level. In addition you will be provided with hundreds of practice problems all targeted specifically for you. Click on the picture below for more information about these books.

If you liked this article, please share it with your Facebook friends:

I’ll see you tomorrow…

Newer posts

Older posts

$500 New SAT Math Problems$

500 New SAT Math Problems
Just 19.99 on Amazon

A Trick For Free Two Day Shipping

SAT and SAT Subject Test Dates

Increasing Your SAT Math Score from 750 to 800

SAT Math Practice Problems

Hard ACT Math Probability Problem with Solution

Level 5 Probability

More ACT Math Problems with Explanations

Hard ACT Math Probability Problem

Level 5 Probability

More ACT Math Problems with Explanations

ACT Math Number Theory Problem with Solutions

Level 3 Number Theory

More ACT Math Problems with Explanations

ACT Math Number Theory Problem

Level 3 Number Theory

More ACT Math Problems with Explanations

Breakdown of the SAT Math Sections

“Calculator Allowed” Section

“No Calculator” Section

Get Eight Books for the Price of Three

AP Calculus BC Problem with Solution – Arc Length

Level 2 Arc Length

More AP Calculus Problems with Explanations

AP Calculus BC Problem- Arc Length

Level 2 Arc Length

More AP Calculus Problems with Explanations

The Differences Between the PSAT and the SAT

Test Length and Structure

Scoring

Final Note

500 New SAT Math Problems Just 19.99 on Amazon

A Trick For Free Two Day Shipping

SAT and SAT Subject Test Dates

Increasing Your SAT Math Score from 750 to 800

SAT Math Practice Problems

Hard ACT Math Probability Problem with Solution

Level 5 Probability

More ACT Math Problems with Explanations

Hard ACT Math Probability Problem

Level 5 Probability

More ACT Math Problems with Explanations

ACT Math Number Theory Problem with Solutions

Level 3 Number Theory

More ACT Math Problems with Explanations

ACT Math Number Theory Problem

Level 3 Number Theory

More ACT Math Problems with Explanations

Breakdown of the SAT Math Sections

“Calculator Allowed” Section

“No Calculator” Section

Get Eight Books for the Price of Three

AP Calculus BC Problem with Solution – Arc Length

Level 2 Arc Length

More AP Calculus Problems with Explanations

AP Calculus BC Problem- Arc Length

Level 2 Arc Length

More AP Calculus Problems with Explanations

The Differences Between the PSAT and the SAT

Test Length and Structure

Scoring

Final Note

500 New SAT Math Problems
Just 19.99 on Amazon