500 New SAT Math Problems
Just 19.99 on Amazon

Hi everyone! The latest edition of 500 New SAT Math Problems is now available in paperback from Amazon. This edition just has been modified from the previous edition to account for the changes on the Digital SAT.

The paperback is now on sale on Amazon for only $19.99. Note that once the sale ends (by the end of today), the price of this book will go up to $42.99. 

The promotion has ended. Thanks to everyone who participated. The book is now available at its regular price here: 500 New SAT Math Problems

If you have any questions, feel free to contact me at steve@SATPrepGet800.com 

Thank you all for your continued support!

A Trick For Free Two Day Shipping

I would like to finish this post with a little trick you can use to get free 2 day shipping on any of the books you decide to purchase without making any additional purchases. If you have never used Amazon Prime you can sign up for a free month using the following link.

Sign Up For Amazon Prime For Free

If you have already had a free trial of Amazon Prime you can simply open up a new Amazon account to get a new free trial. It just takes a few minutes! You will need to use a different email address than the one you usually use.

This next part is very important! After you finish your transaction, go to your Account, select “Manage my prime membership,” and turn off the recurring billing. This way in a month’s time Amazon will not start charging you for the service.

After shutting off the recurring billing you will still continue to receive the benefit of free 2 day shipping for one month. This means that as long as you use this new Amazon account for your purchases you can do all of your shopping on Amazon for the next month without having to worry about placing minimum orders to get free shipping.

Just be aware that certain products from outside sellers do not always qualify for free shipping, so please always check over your bill carefully before you check out.

Well I hope you decide to take advantage of this very special offer, or at the very least I hope you will benefit from my Amazon “free 2 day shipping trick.” Here is the link one more time:

Sign Up For Amazon Prime For Free

If you think your friends might be interested in this special offer, please share it with them on Facebook:

Thank you all for your continued support!

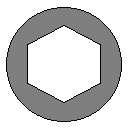Hard Geometry Problem for the SAT

Today I would like to provide an example of a hard geometry problem that could show up on the SAT.

Level 4 – Geometry

The head of a copper “hexagon head screw bolt” (one cross section of which is shown above) has the shape of a cylinder with a hole shaped like a regular hexagon. The cylindrical head is 2 cm thick with a base diameter of 3 cm. The hexagonal hole is only half the thickness of the entire head, and each side of a hexagonal cross section has a length of  1 cm.­ Given that the density of copper is 8.96 grams per cubic cm, and density is mass divided by volume, find the mass of the head to the nearest gram.

Please feel free to post your solutions or attempted solutions in the comments below and we can discuss the best way to solve the problem. I will post solutions to this problem next week.

More Hard SAT Math Practice Problems

If you are preparing for the SAT you may want to check out the Get 800 collection of SAT math books.

If you think your friends would like to try this problem, please share:

Speak to you soon!

New SAT Prep Book

Today I would like to announce the release of the New SAT Prep Book for Reading, Writing and Math Mastery. This book contains all of the content from the math and verbal books for the revised SAT that I have previously released.

The paperback is now on sale on Amazon for only $8.14. This sale may run for just a few hours (until 12 PM), and once the sale ends the price will go up to $34.99. If you want to take advantage of the sale price I strongly recommend you purchase the book right away.

The sale has ended. I will however still honor the promotions below until the end of the month.

Click the following link to get to the book’s Amazon page: New SAT Prep Book

Note that this book is for the revised SAT beginning March, 2016.

As an additional incentive to purchase this book today, I will also give you another book for FREE as a downloadable PDF file. You can choose ANY of my other books. So if you would like a different book, go ahead and purchase this one (as soon as it is available), forward me your Amazon confirmation email and let me know which of my books you would like for free. You will be provided with a link to download your additional free book. This offer is available until the end of today (October 8, 2015).

Take a look at my product page to see all of my books: Get 800 Product Page

I am also making the following promotion available until October 30, 2015: if you buy any 3 of my books in paperback, you get all 6 of the following SAT math prep books for free.

A Trick For Free Two Day Shipping

I would like to finish this post with a little trick you can use to get free 2 day shipping on any of the books you decide to purchase without making any additional purchases. If you have never used Amazon Prime you can sign up for a free month using the following link.

Sign Up For Amazon Prime For Free

If you have already had a free trial of Amazon Prime you can simply open up a new Amazon account to get a new free trial. It just takes a few minutes! You will need to use a different email address than the one you usually use.

This next part is very important! After you finish your transaction, go to your Account, select “Manage my prime membership,” and turn off the recurring billing. This way in a month’s time Amazon will not start charging you for the service.

After shutting off the recurring billing you will still continue to receive the benefit of free 2 day shipping for one month. This means that as long as you use this new Amazon account for your purchases you can do all of your shopping on Amazon for the next month without having to worry about placing minimum orders to get free shipping.

Just be aware that certain products from outside sellers do not always qualify for free shipping, so please always check over your bill carefully before you check out.

Well I hope you decide to take advantage of this very special offer, or at the very least I hope you will benefit from my Amazon “free 2 day shipping trick.” Here is the link one more time:

Sign Up For Amazon Prime For Free

If you think your friends might be interested in this special offer, please share it with them on Facebook:

Thank you all for your continued support!

New SAT Prep Book for Reading, Writing and Math Mastery

Today I am happy to announce that the New SAT Prep Book for Reading, Writing and Math Mastery  will be available this week. This book will prepare you for the revised SAT beginning March, 2016 (as well as the PSAT being administered in October, 2015).

This book will be released on Amazon  at a promotional price of about $8.00 for a few hours.

Once the sale ends the price will go up to $39.99. If you want to take advantage of the sale price I strongly recommend that you get on the notification list.

If you want to be added to my email notification list for this and future promotions, send an email to steve@SATPrepGet800.com with “Notify me” written in the subject line.

In the meantime, you can take a look at my product page to see all of the other books from the Get 800 collection: Get 800 Product Page

If you think your friends might be interested in this special offer, please share it with them on Facebook:

Thank you all for your continued support!

Heart of Algebra Problem

Last week I gave you a Level 5 “Heart of Algebra” problem for the revised SAT to try. Today I will provide a solution for this problem. If you have not yet attempted the problem go back and take a look at it first so you can try it on your own. Here is the link: Hard Algebra Problem for the Revised SAT

Level 5 – Heart of Algebra

Here is the problem once again followed by several solutions:

3x – 7y = 12
kx + 21y = -35

For which of the following values of k will the system of equations above have no solution?

(A)  9
(B)  3
(C) -3
(D) -9

Solution: The system of equations

ax + by = c
dx + ey = f

has no solution if a/d = b/e ≠ c/f. So we solve the equation 3/k = -7/21.  Cross multiplying yields 63 = -7k  so that k = 63/-7 = -9, choice (D).

Note: In this problem b/e ≠ c/f. Indeed, -7/21 ≠ 12/-35. This guarantees that the system of equations has no solution instead of infinitely many solutions.

* Quick solution: We multiply -7 by -3 to get 21. So we have k = (3)(-3) = -9, choice (D).

Note: The general form of an equation of a line is ax + by = c where a, b, and c are real numbers. More information about this type of equation can be found in New SAT Math Problems arranged by Topic and Difficulty Level.

Feel free to add your own solutions to the comments.

More Hard SAT Math Practice Problems

For many more hard SAT math problems like these, each with several fully explained solutions, check out New SAT Math Problems arranged by Topic and Difficulty Level. Click on the picture below for more information about this book.

If you think your friends would like to try this problem, please share:

Speak to you soon!

Hard Heart of Algebra Problem

Today I would like to provide an example of a hard “Heart of Algebra” problem that could show up on the revised SAT.

Level 5 – Heart of Algebra

3x – 7y = 12
kx + 21y = -35

For which of the following values of k will the system of equations above have no solution?

(A)  9
(B)  3
(C) -3
(D) -9

Please feel free to post your solutions or attempted solutions in the comments below and we can discuss the best way to solve the problem. I will post solutions to this problem next week.

More Hard SAT Math Practice Problems

For many more hard SAT math problems like these, each with several fully explained solutions, check out New SAT Math Problems arranged by Topic and Difficulty Level. Click on the picture below for more information about this book.

If you think your friends would like to try this problem, please share:

Speak to you soon!

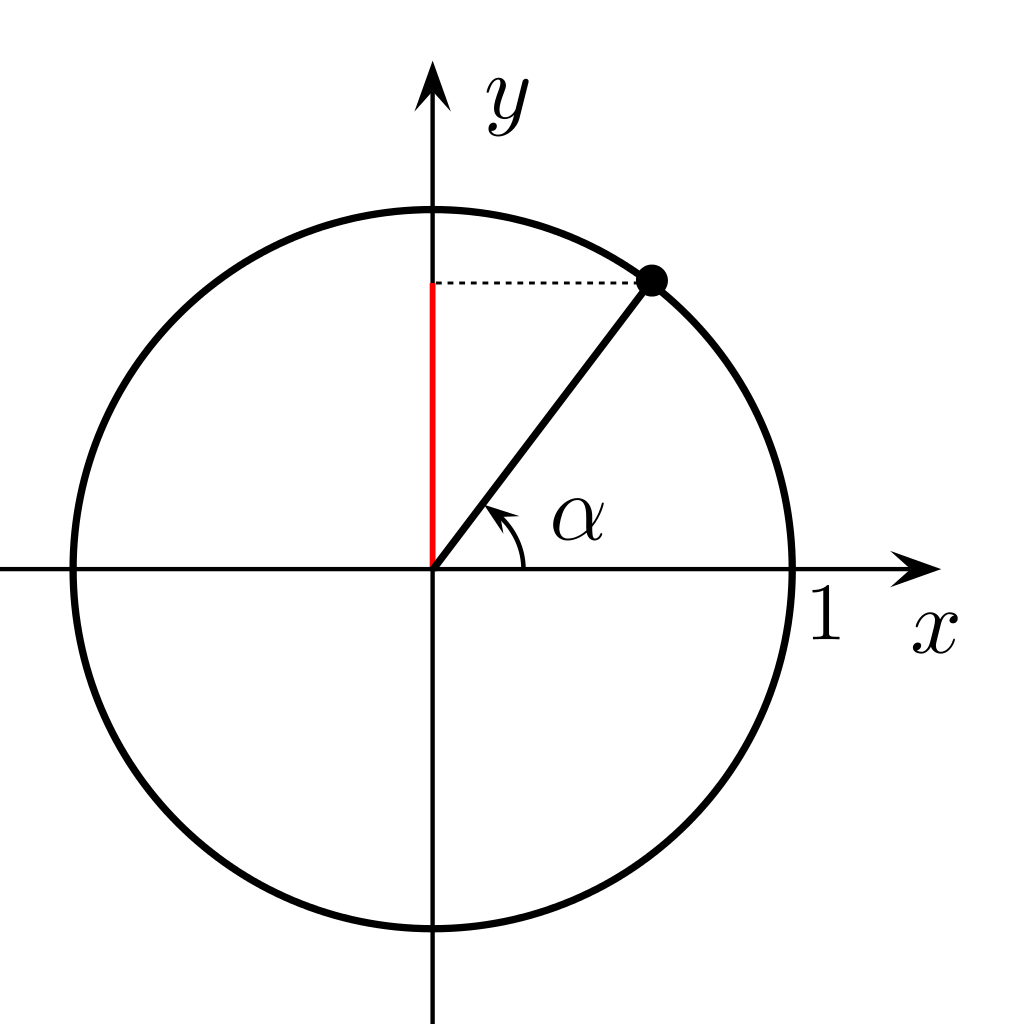Hard Trigonometry Problem

Last week I gave you a Level 5 trigonometry problem for the revised SAT to try. Today I will provide a solution for this problem. If you have not yet attempted the problem go back and take a look at it first so you can try it on your own. Here is the link: Hard Trigonometry Problem for the Revised SAT

Level 5 – Trigonometry

Here is the problem once again followed by a solution:

It is given that cos x = k, where π < x < 3π/2 and k is the radian measure of an angle. If cos z = –k, which of the following could not be the value of  z ?

(A) x – π
(B) π – x
(C) 2π – x
(D) 3π – x

Solution using coterminal angles and a negative identity: 

cos(2π – x) = cos(x – 2π) = cos x = k ≠ –k.

So 2π – x  could not be the value of z, choice (C).

Notes: (1) For the first equality we used the negative identity

cos(–A) = cos A

(see problem 104 in New SAT Math Problems), together with the fact that

x – 2π = –(2π – x) .

(2) In general we have a – b = -(b – a) . To see this simply distribute:

-(b – a) = –b + a = a – b. 

(3) Using notes (1) and (2) together, we have

cos(2π – x) = cos(-(x – 2π)) = cos(x – 2π).

(4) Several more notes giving more insight into this solution can be found in New SAT Math Problems arranged by Topic and Difficulty Level.

For additional solutions to this problem such as using the cosine difference identity and using the unit circle check out New SAT Math Problems arranged by Topic and Difficulty Level.

Feel free to add your own solutions to the comments.

More Hard SAT Math Practice Problems

For many more hard SAT math problems like these, each with several fully explained solutions, check out New SAT Math Problems arranged by Topic and Difficulty Level. Click on the picture below for more information about this book.

If you think your friends would like to try this problem, please share:

Speak to you soon!

Hard Trigonometry Problem

The revised SAT will contain a very small number of trigonometry questions. Today I would like to provide an example of a particularly hard trigonometry problem that could show up.

Level 5 – Trigonometry

It is given that cos x = k, where π < x < 3π/2 and k is the radian measure of an angle. If cos z = –k, which of the following could not be the value of  z ?

(A) x – π
(B) π – x
(C) 2π – x
(D) 3π – x

Please feel free to post your solutions or attempted solutions in the comments below and we can discuss the best way to solve the problem. I will post several solutions to this problem next week.

More Hard SAT Math Practice Problems

For many more hard SAT math problems like these, each with several fully explained solutions, check out New SAT Math Problems arranged by Topic and Difficulty Level. Click on the picture below for more information about this book.

If you think your friends would like to try this problem, please share:

Speak to you soon!

Hard SAT Math Problem

Last week I gave you a Level 5 SAT math problem for the revised SAT to try. Today I will provide solutions for this problem. If you have not yet attempted the problem go back and take a look at it first so you can try it on your own. Here is the link: Hard SAT Math Problem for the Revised Exam

Level 5 – Passport to Advanced Math

Here is the problem once again followed by several solutions:

If x² – 8x = 209 and x < 0, what is the value of |x + 3| ?

Algebraic solution: Let’s attempt to factor the equation. We begin by subtracting 209 from each side to get x² – 8x – 209 = 0. We now factor the left hand side to get (x – 19)(x + 11) = 0.

So x = 19 or x = -11. Since we are given x < 0, we use x = -11.

Finally we have |x +3| = |-11 + 3| = |-8| = 8.

Notes: (1) There are several ways to solve a quadratic equation. A few are by (i) factoring, (ii) completing the square, (iii) the quadratic formula, (iv) guessing and checking, (v) creating a table of values in your calculator, (vi) using the graphing features of your calculator.

(2) Whenever you solve a quadratic equation by factoring or by using the quadratic formula, you need to bring everything over to one side of the equation first, leaving 0 on the other side.

(3) It can seem very difficult at first to find the factors of 209, but the following trick can help you find the factors a little easier. Since we have 15² = 225 > 209, it follows that if 209 can be factored, then it has a factor less than 15. Furthermore, since every positive integer can be factored as a product of primes, it follows that we need only check prime numbers less than 15. So we can simply check 209 for divisibility by 2, 3, 5, 7, 11, and 13.

We can use standard divisibility tricks to eliminate 2, 3, and 5 right away (if you don’t know these tricks you can learn about them here: Divisibility Tricks). You may want to try 11 next since it is pretty easy to divide by 11. In this case we have 209/11 = 19. So 209 = 11 ∙ 19.

If we happen to be allowed to use a calculator for this problem, then we could divide 209 by these numbers very quickly.

(4) Several more notes giving more insight into this solution can be found in New SAT Math Problems arranged by Topic and Difficulty Level.

Solution by guessing and checking: If we are allowed to use our calculator, there are several other ways to solve the given equation for . One way is to simply guess and check negative values for x. For example if we substitute -3 in for x on the left hand side of the equation we get (-3)^2 – 8(-3) = 33, which is too small. So let’s try x = -8 next: (-8)^2 – 8(-8) = 128 . This is still too small, but we’re heading in the right direction. We try x = -11 to get (-11)^2 – 8(-11) = 209.

It works. So x = -11  and we have |x +3| = |-11 + 3| = |-8| = 8.

For additional solutions to this problem such as completing the square, using the quadratic formula, creating a table of values, and by graphing check out New SAT Math Problems arranged by Topic and Difficulty Level. 

Feel free to add your own solutions to the comments. 

More Hard SAT Math Practice Problems

For many more hard SAT math problems like these, each with several fully explained solutions, check out New SAT Math Problems arranged by Topic and Difficulty Level. Click on the picture below for more information about this book.

If you think your friends would like to try these problems, please share:

Speak to you soon!

Hard SAT Math Problem

Hello everybody. I think it’s time for an example of a difficult math problem that may show up on the new SAT. This one is a Level 5 free response question (or grid-in).

Level 5 – Passport to Advanced Math

If x² – 8x = 209 and x < 0, what is the value of |x + 3| ?

Please feel free to post your solutions or attempted solutions in the comments below and we can discuss the best way to solve the problem. I will post several solutions to this problem next week.

More Hard SAT Math Practice Problems

For many more hard SAT math problems like these, each with several fully explained solutions, check out New SAT Math Problems arranged by Topic and Difficulty Level. Click on the picture below for more information about this book.

If you think your friends would like to try these problems, please share:

Speak to you soon!

Polynomial and Rational Functions

Today I have uploaded a video to my YouTube channel on Polynomial and Rational Functions. You can visit the YouTube page here: ACT Prep – Polynomial and Ratonal Functions

For your convenience I have also embedded this video below:

 

If you liked this video, please share it with your Facebook friends:

Until next time…