500 New SAT Math Problems
Just 19.99 on Amazon

Hi everyone! The latest edition of 500 New SAT Math Problems is now available in paperback from Amazon. This edition just has been modified from the previous edition to account for the changes on the Digital SAT.

The paperback is now on sale on Amazon for only $19.99. Note that once the sale ends (by the end of today), the price of this book will go up to $42.99. 

The promotion has ended. Thanks to everyone who participated. The book is now available at its regular price here: 500 New SAT Math Problems

If you have any questions, feel free to contact me at steve@SATPrepGet800.com 

Thank you all for your continued support!

A Trick For Free Two Day Shipping

I would like to finish this post with a little trick you can use to get free 2 day shipping on any of the books you decide to purchase without making any additional purchases. If you have never used Amazon Prime you can sign up for a free month using the following link.

Sign Up For Amazon Prime For Free

If you have already had a free trial of Amazon Prime you can simply open up a new Amazon account to get a new free trial. It just takes a few minutes! You will need to use a different email address than the one you usually use.

This next part is very important! After you finish your transaction, go to your Account, select “Manage my prime membership,” and turn off the recurring billing. This way in a month’s time Amazon will not start charging you for the service.

After shutting off the recurring billing you will still continue to receive the benefit of free 2 day shipping for one month. This means that as long as you use this new Amazon account for your purchases you can do all of your shopping on Amazon for the next month without having to worry about placing minimum orders to get free shipping.

Just be aware that certain products from outside sellers do not always qualify for free shipping, so please always check over your bill carefully before you check out.

Well I hope you decide to take advantage of this very special offer, or at the very least I hope you will benefit from my Amazon “free 2 day shipping trick.” Here is the link one more time:

Sign Up For Amazon Prime For Free

If you think your friends might be interested in this special offer, please share it with them on Facebook:

Thank you all for your continued support!

Hello everyone. This post is mostly for more advanced students that are trying to get an 800 in SAT math (or close to it). That said, the strategy I will be describing here is actually very easy to apply. So even if you are not going for an 800, it will not take too much effort to learn this technique.

Today I would like to talk about Xiggi’s formula.

First of all, some of you may want to know who Xiggi is. Well Xiggi is a frequent poster on the SAT preparation forum of College Confidential.  If you are preparing for the SAT I highly recommend that you utilize this forum as a resource.

The formula I will be discussing in this post is actually called the harmonic mean formula, but on the College Confidential site we all call it Xiggi’s formula. The reason for this is because Xiggi has become well known for helping students on the forum to solve this particular type of problem.

So when should one use Xiggi’s formula? Well, Xiggi’s formula can be used to find an average rate when two individual rates for the same distance are known.

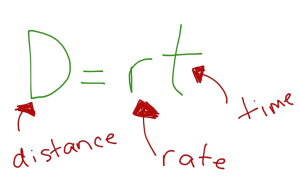
Here is the formula:

Important notes:  (1) Xiggi’s Formula only works when the two distances are the same.

(2) Even though the distance is often given in these types of problems, note that the formula does not use the distance. It uses only the two rates.

(3) For these types of SAT problems, the words “rate” and “speed” are interchangeable.

Here is an example of a problem where Xiggi’s formula is the best way to go:

An elephant traveled 7 miles at an average rate of 4 miles per hour and then traveled the next 7 miles at an average rate of 1 mile per hour. What was the average speed, in miles per hour, of the elephant for the 14 miles?

Let’s solve this problem using Xiggi’s formula. We have

Just to compare, let’s also solve this problem in the more traditional way – by writing out a “distance = rate · time” chart.

  ________________   _Distance                  Rate                       Time
1st part of trip                 7                            4                          7/4
2nd part of trip               7                            1                          7/1 = 7
14                                                        8.75

Note that we computed the times by using “distance = rate · time” in the form

Finally, we use the formula in the form

Note: To get the total distance we add the two distances, and to get the total time we add the two times. Be careful – this doesn’t work for rates!

SAT math problems of this type are always Level 5 problems. But if you know Xiggi’s formula, you can solve this type of question in just a few seconds. So in my opinion, it is well worth committing this one to memory.

Here is another SAT math question where Xiggi’s formula can be useful. Give this one a try on your own.

Jason ran a race of 1600 meters in two laps of equal distance. His average speeds for the first and second laps were 11 meters per second and 7 meters per second, respectively. What was his average speed for the entire race, in meters per second?

See if you can solve this problem using Xiggi’s formula. And then also try to solve it with a “distance = rate · time” chart.

I will provide both solutions in next week’s post. But feel free to leave your own solutions in the comments.

Remember that Xiggi’s formula should only be used when the two distances are the same! Should it ALWAYS be used when the distances are the same? We will address this question in next week’s post where we will look at distance, rate, time problems where Xiggi’s formula is not as useful.

Until then, I will leave you with the following “Challenge Problem.”

Use the formula d = rt to derive Xiggi’s formula.

The first three students to provide a complete and correct solution will receive a free downloadable copy of one of my books. You can post the solution in the comments below or on my Facebook wall:

www.facebook.com/SATPrepGet800

Hello everyone.

Two weeks ago I posted a video teaching a very basic but important SAT math strategy – Starting With Choice (C).

Very often when plugging in answer choices, choice (C) is the best place to start. But there are some exceptions. Today’s video will talk about those exceptions. You can see the blog post that I have already written on these two subjects here: Starting With Choice (C) – A Basic SAT Math Strategy

But I have also uploaded a video to my YouTube channel describing this strategy in detail. You can visit the YouTube page here: Plugging In Answer Choices – When Not To Start With Choice (C)

For your convenience I have also embedded both of these videos below:

 

 

Hello everyone.

As some of you know, my best-selling book on Amazon is 320 SAT Math Problems arranged by Topic and Difficulty Level.

One thing that I have noticed is that there are many students that purchase this book that really only want to work on the most difficult problems. It is because of this that I decided to release an abridged version of this book at a lower price.

This new abridged version of the 320 Problems book is called the SAT Prep Book of Advanced Math Problems.

This book contains 192 Level 3, 4, and 5 problems that are uniformly distributed across all topics and each of these three difficulty levels. This book is ideal for students scoring over a 600 that do not feel the need to practice additional Level 1 and 2 Problems. Any students that wish to practice the lower level problems as well should consider purchasing 320 SAT Math Problems arranged by Topic and Difficulty Level instead of this book.

Also remember that I have several other SAT math prep books available. In order for you to decide which of them is the best for you I recommend that you read the following blog post: Which SAT Math Prep Book Should I Use?

And please don’t forget to post any SAT math questions that you are having trouble with on my Facebook wall.

Best of luck to all of you that are taking the SAT. I’ll see you next week with some more great SAT math strategies…

Hello everyone.

There are some very basic SAT math strategies that every high school student should know. One such strategy is “plugging in answer choices.” I’ve already written a blog post on this subject here: Starting With Choice (C) – A Basic SAT Math Strategy

I have also just uploaded a video to my YouTube channel describing this strategy in detail. You can visit the YouTube page here: Start With Choice (C) – An Important Math Strategy

The video is also embedded below for your convenience:

 

How are you today?

Many of you have already seen my article on avoiding careless errors in sat math. This particular article I feel is one of the more important messages I give for students that are preparing for the SAT.

It is so important that I have actually taken the time to create a video for you.

If you want watch the video on YouTube, then please visit the following link: how to avoid careless mistakes in SAT math.

I have also embedded the video here for you. Enjoy!

 

Recognizing Blocks

Today, let’s discuss an SAT math strategy I like to call “Recognizing Blocks.”

First, let me give my definition of a block: A block is an algebraic expression that appears more than once in a given problem.

Very often in SAT problems a block can be treated just like a variable. In particular, blocks should usually not be manipulated—treat them as a single unit.

This is simple in theory. Let’s try it with a Level 2 SAT math problem:

Examples:

1. If 3x – 5y = 7 , what is the value of 6(3x − 5y) ?
               (A)   50
               (B)   42
               (C)   36
               (D)   30
               (E)   4

In this example there is a block of 3x – 5y. Let’s look at the following seemingly easier problem:

If a = 7, what is the value of 6a?

The answer to this problem is (6)(7) = 42.

But this is essentially the same problem as the one we were given. We just replaced the block by the variable a. So the answer to the original question is also 42, choice (B).

You can be really sharp by doing this problem in your head in just a few seconds: (6)(7) = 42. choice (B).

Easy, right?

Here is a more difficult problem for you:

2. If b = 7(u + v + x + y +z)/5, then in terms of b what is the average (arithmetic mean) of u, v, x, y, and z?

               (A)   b/35
               (B)   b/7
               (C)   b/5
               (D)   5b
               (E)   7b

In this example there is a block of a = (u + v + x + y + z)/5 which is precisely the average of u, v, x, y, and z. So solving this problem is the same as solving the following equation for a.

b = 7a

To solve this equation for a we will simply divide both of the sides by 7 to get a = b/7, choice (B).

These problems as relatively straightforward to solve as long as you treat all blocks as variables and do not try to manipulate them.

There are several examples of problems where this math strategy can be used in the College Board’s Blue Book, but if you want to get even more practice then take a look at my 28 SAT Math Lessons Series. Click on the picture below for more information about these books.

If you liked this article, please share it with your Facebook friends:

And if you have any questions on this topic, then please post them on my Facebook wall:

Hi all,

A few months back I explained how each of my books has been designed for a specific reason. In this post I would like to give a brief description about each of the books to help you decide which one (or ones) is right for you.

SAT Prep Official Study Guide Math Companion – This contains my solutions to all problems in the Blue Book tests (1-10 and DVD). Most problems are solved in several different ways using methods that are much simpler and more efficient than the College Board’s solutions.

28 SAT Math Lessons To Improve Your Score In One Month (Advanced Course) – This is a one month course designed for students currently scoring over 600 in SAT math. If used properly by these students, a perfect 800 or near perfect score can be achieved. Please note that this book contains well over 300 problems, most of which are level 4 and 5. Some of these problems are extremely rare and quirky in nature, but everything has been included for the student that is going for a perfect score.

28 SAT Math Lessons To Improve Your Score In One Month (Intermediate Course) – This is a one month course designed for students scoring between 500 and 600 in SAT math. It contains all the most important strategies, and problem types designed to take students’ scores closer to a 700. This course takes all the guesswork out of what to study.

28 SAT Math Lessons To Improve Your Score In One Month (Beginner Course) – This is a one month course designed for students scoring below 500 in SAT math. This is a very low intensity course designed to get unmotivated or frustrated students with a weak mathematical background to begin solving SAT math problems. It contains only the most basic strategies and problem types designed to take students’ scores closer to a 600. This course takes all the guesswork out of what to study.

320 SAT Math Problems Arranged By Topic And Difficulty Level – As the title suggests, this book has problems arranged by topic and difficulty level. Note that the problems in this book are uniformly distributed among all levels and topics. If you are looking for a book that focuses on only difficult SAT math problems, then this is NOT the right book for you. The very advanced student will get much more value out of the Advanced Course 28 Lessons book.

The 32 Most Effective SAT Math Strategies – In here you will find all the most important and significant tips, tricks and tactics to solve problems you will face in SAT math. Internalize these strategies and you will be able to answer more SAT math problems in less time.

I have actually updated all of the books’ product pages with this information, so I hope this make things a bit clearer for those of you that are unsure which of my 6 books might be best for you.

Common SAT Math Strategies

Welcome back to the GET 800 blog – The only place you need to visit for SAT math hints, tips and tactics!

I have been asked some excellent questions on my Facebook page lately. And today I will address one of these questions that had been asked only a few days ago. If you have any SAT math related query that you want answered, simply post it up there and I will come back with an answer ASAP.

There are three very basic SAT math strategies that every high school student should know: Plugging In Answer Choices, Taking Guesses, and Picking Numbers. Take a look at these links before you continue with this message, as the information below will make more sense after a review of these strategies.

Sometimes students get confused about when to use which of these strategies. Although there are no definite rules I can give you that will work 100 percent of the time, today I will provide you with some general guidelines.

Plugging In

Let’s begin with the strategy of plugging in answer choices:

This strategy can only be used for multiple choice questions. You simply plug each answer choice into the question until you find the one works. It is always best to start with choice (C) as your first attempt, unless there is a specific reason not to. An example of such a reason would be that the word “least” appears in the problem. In this case start with the smallest answer choice (which will usually be choice (A) or (E)). Similarly, if the word greatest appears in the problem, start with the largest answer choice.

Plugging in can be used on problems from every topic and difficulty level. It’s often a great way to avoid having to perform messy algebra.

This strategy is most useful when the last part of the question says “What is_____” where the blank contains a single quantity.

Here are some examples:

(1) What is the value of x?
(2) What is the length of the original rectangle?
(3) What is the second number in the list?
(4) Which of the following is a perfect cube?
(5) What is the least such value of x?

For the first four of these examples you would start with choice (C). For the fifth example you should start with the smallest answer choice.

This strategy is generally NOT useful when you are asked to find a more complicated expression. For example, do not try to plug in answer choices if the question ends with “What is the value of x + y?” In this case you will probably want to use the strategy of “Taking a guess,” or possibly “Picking numbers.”

During your SAT math practice sessions, you should try to apply this strategy on every multiple choice question. The more you attempt to use it, the easier it will be to detect when it can be applied.

Here is a straightforward example of a Level 1 Number Theory problem where “starting with choice (C)” is a useful strategy.

Three consecutive integers are listed in increasing order. If their sum is 138, what is the second integer in the list?

(A) 45
(B) 46
(C) 47
(D) 48
(E) 49

I suggest you try to solve this problem in 3 ways:

• by starting with choice (C)
• algebraically
• using the fact that in a list of consecutive integers, the arithmetic mean is equal to the median.

And here is a Level 4 Geometry problem where this strategy is effective:

The sum of the areas of two squares is 85. If the sides of both squares have integer lengths, what is the least possible value for the length of a side of the smaller square?

(A) 1
(B) 2
(C) 6
(D) 7
(E) 9

You should solve this problem by starting with choice (A).

There are more examples in my original blog post titled Starting With Choice (C) – A Basic SAT Math Strategy.

Taking Guesses

Next let’s talk about the strategy of taking a guess:

This strategy is similar to plugging in, except with this one you are not using the answer choices. The reason you are not using the answer choices is either because it is a grid in problem (so there are no answer choices), or the question is asking for a more complicated expression such as “the value of x + y,” or “the perimeter of a geometric figure. In the first case you may want to take a guess for x or y, and in the second case you may want to take a guess for the width of a side of the figure. For examples of applying this strategy see my original blog post titled Basic SAT Math Strategy – Take A Guess. For a more difficult example, see my blog post titled Taking a Guess – A Further Example Of How To Use This Technique.

Picking Numbers

And finally, let’s talk about the strategy of picking numbers:

This strategy is applied by choosing specific values for the unknown quantities in the problem. A new problem is formed that is easier to solve. After solving this easier problem, you must then plug the specific values you have picked into every answer choice, and eliminate any answer choices that do not come out correct. If more than one answer choice has not been eliminated, you can try picking new numbers to eliminate more choices.

Here are some occasions when the strategy of picking numbers will usually work:

• When one or more variables appear in the answer choices.
• When the word “percent” is in the problem (pick 100).
• When there is a variable in the problem, and it is implied that the answer does not depend on the value of the variable.

Here is an Algebra problem where “Picking numbers” can be used.

Which of the following is equal to (x + 66)/22 ?

(A) (x+33)/11
(B) x+3
(C) 3x
(D) x/22+3
(E) (x+3)/11

Note that you usually want to avoid picking numbers that are too simple. For example, if you choose x = 0, then the answer to the question becomes 3. Now, if you plug a 0 in for x into every answer choice you get the following:

(A) 3
(B) 3
(C) 0
(D) 3
(E) 3/11

Note that (A), (B), and (D) are all correct.

See if you can solve this problem by picking a better number. You may also want to try to solve it algebraically.

For examples of applying this strategy see my original blog post on Picking Numbers.

Picking Numbers In Percent Problems

If the word “percent” appears in a problem it’s usually a great idea to choose the number 100. This often works even when there is no variable in the problem.

For examples of picking numbers in percent problems, see my blog post titled Picking A Number To Solve Percent Problems.

Summary

To summarize, here are some general guidelines for choosing which strategy to use:

• If the answer choices are all numbers, and you are being asked for a simple quantity, try plugging in first.
• If the answer choices are all numbers, and you are being asked for a more complicated quantity, try taking a guess.
• If the answer choices contain variables, try picking a number.
• Any question that mentions percents, pick the number 100.
• In grid in questions with variables, try taking a guess.

There is a lot of information in this blog post with links to many of my other posts, so take your time to read and digest the information. As always, the best way to internalize the concepts above is by practice. So get your Blue Book and get practicing! If you would like additional practice, check out my 28 SAT Math Lessons Series. Click on the picture below for more information about these books.

If you liked this article, please share it with your Facebook friends:

And if you have any questions on this topic, then please post them on my Facebook wall:

I will answer your questions right away.

Hello everyone.

If you have been reading this blog, then you are probably familiar with what the SAT is. In case you are not, here is a link to one of my articles that gives detailed information on this: Overview of the Math Sections of the SAT

One thing that I have not yet talked about is the PSAT.

First, did you know that the “P” in PSAT stands for preliminary? Most people incorrectly think that it stands for practice. 

Also, almost twice the number of test takers take the PSAT compared to the SAT. This is because the PSAT determines eligibility and qualification for the National Merit Scholarship Program.

In addition to College Board Blue Book tests, almost all of my students take the PSAT to gauge how well they are going to do on the SAT. There are a few major differences between the PSAT and the SAT and this post will shed some light on these differences.

Test Length and Structure

The PSAT consists of 5 sections. There are two 25 minute reading sections, two 25 minute math sections, and one 30 minute writing section. This gives a total testing time of 2(25) + 2(25) + 30 = 130 minutes, or 2 hours and 10 minutes. If we add in the 5 minute break after section 2 and the 1 minute break after section 4 we get a total time of 2 hours and 16 minutes from the beginning to the end of the test.

The SAT is much, much, much longer than the PSAT. It consists of 10 sections – that’s right, double the amount that is on the PSAT. There is one 25 minute essay, one 25 minute writing section, one 10 minute writing section, two 25 minute reading sections, one 20 minute reading section, two 25 minute math sections, one 20 minute math section, and one 25 minute experimental section which may be reading or math. This gives a total testing time of 7(25) + 2(20) + 10 = 225 minutes, or 3 hours and 45 minutes. If we add in the three 5 minute breaks, we get a total time of 4 hours from the beginning to the end of the test.

So although the PSAT is supposed to prepare you for the SAT, one thing that it definitely does NOT prepare you for is the amount of endurance necessary to get through the SAT. The SAT is the first, and maybe only test that you will ever take that requires this kind of time commitment. Fatigue alone can be responsible for causing a decrease in score from your PSAT to your SAT.

Scoring

The PSAT is graded out of 240 points with each of reading, writing and math consisting of a total of 80 points.

The SAT is graded out of 2400 points with each of reading, writing and math consisting of a total of 800 points.

So you can essentially convert your PSAT score to the corresponding SAT score by tacking on an extra zero at the end.

This is not entirely accurate however.

In other words, suppose a student were to score a 50 in math on their PSAT. Does that mean that if they had taken the SAT instead that day that they would have scored a 500? The answer in general is no – they most likely would have scored a bit lower than that. A rough estimate is about 5% lower.

So, in other words, after taking your PSAT, to get your approximate SAT score tack on a zero to the end, and take away 5 percent. This is equivalent to multiplying your PSAT score by 9.5.

For example, a student that scored 170 on their PSAT would have received approximately 170(9.5) = 1615 on their SAT. Of course 1615 is not actually a possible SAT score, but again, this is just a rough estimate.

Note that there is no statistical data to support the accuracy of this computation. After all, nobody takes a PSAT and an SAT on the same day. This is just my own personal way of estimating what I believe is happening.

Other than “the missing zero” all other aspects of scoring the PSAT and the SAT are the same. Multiple choice questions and grid ins are handled the same way, and the raw score is computed in the same fashion. For more details on how this is done, see this article titled Overview of the Math Sections of the SAT.

Just one comment on the scaled score – although for most students the scaled score is handled almost identically on both the PSAT and SAT, the strongest students may be penalized a bit more heavily on the PSAT. For example just one wrong question on the math section of the PSAT could drop your scaled score down 5 points. This could really hurt if you are applying for a National Merit Scholarship.

Material Covered

Almost all of the topics covered on the PSAT and SAT are identical. The only exception is a few math concepts that are left out of the PSAT. These concepts are not put on the PSAT because a large percentage of students have not yet covered these topics in school by October of their junior year (which is when most students take the PSAT).

The topics that are left off of the PSAT mostly pertain to functions and their graphs. These are topics that are generally introduced in Algebra 2.

The SAT also has one type of section that the PSAT does not – the essay. Students do NOT have to write an essay on the PSAT, but on the SAT this is one of the 10 sections.

Final Note

As mentioned in the section on scoring above, if you decide to take your SAT without any preparation, then it is more likely that your score will go down about 5% from your PSAT than go up. So whether you use a tutor, take a prep course, or prepare on your own, please prepare. With good preparation you can show a significant increase in score from your PSAT to your SAT.

If you do decide to study on your own, I suggest you take a look at my “28 SAT Math Lessons” series. Each book in this series is targeted for students in a specific score range. Get the book for your score range and you will learn all the strategies you need to get to the next score level. In addition you will be provided with hundreds of practice problems all targeted specifically for you. Click on the picture below for more information about these books.

If you liked this article, please share it with your Facebook friends:

I’ll see you next week…