500 New SAT Math Problems
Just 19.99 on Amazon

Hi everyone! The latest edition of 500 New SAT Math Problems is now available in paperback from Amazon. This edition just has been modified from the previous edition to account for the changes on the Digital SAT.

The paperback is now on sale on Amazon for only $19.99. Note that once the sale ends (by the end of today), the price of this book will go up to $42.99. 

The promotion has ended. Thanks to everyone who participated. The book is now available at its regular price here: 500 New SAT Math Problems

If you have any questions, feel free to contact me at steve@SATPrepGet800.com 

Thank you all for your continued support!

A Trick For Free Two Day Shipping

I would like to finish this post with a little trick you can use to get free 2 day shipping on any of the books you decide to purchase without making any additional purchases. If you have never used Amazon Prime you can sign up for a free month using the following link.

Sign Up For Amazon Prime For Free

If you have already had a free trial of Amazon Prime you can simply open up a new Amazon account to get a new free trial. It just takes a few minutes! You will need to use a different email address than the one you usually use.

This next part is very important! After you finish your transaction, go to your Account, select “Manage my prime membership,” and turn off the recurring billing. This way in a month’s time Amazon will not start charging you for the service.

After shutting off the recurring billing you will still continue to receive the benefit of free 2 day shipping for one month. This means that as long as you use this new Amazon account for your purchases you can do all of your shopping on Amazon for the next month without having to worry about placing minimum orders to get free shipping.

Just be aware that certain products from outside sellers do not always qualify for free shipping, so please always check over your bill carefully before you check out.

Well I hope you decide to take advantage of this very special offer, or at the very least I hope you will benefit from my Amazon “free 2 day shipping trick.” Here is the link one more time:

Sign Up For Amazon Prime For Free

If you think your friends might be interested in this special offer, please share it with them on Facebook:

Thank you all for your continued support!

Why Can’t We Divide By Zero?

Two weeks ago, I wrote a post on divisibility tricks – how to quickly recognize if a number is divisible by another number without the use of a calculator. I have had a few questions regarding one of the statements I made concerning division involving zero. The answers to these questions are quite a bit more difficult than anything you will need for the SAT. So instead of adding this information to the previous post, I thought it would be better for me to create a new blog post on this subject.

Whenever I ask a student why we can’t divide by zero I always get strange answers. Most students just restate the question in another way. Some typical answers are “because division by 0 is impossible,” “because my teacher told me I can’t,” or “because my calculator gives an error when I try to do it.”

But none of these “answers” explain anything.

We will clear up this mystery by the end of this post.

Some of the questions we will answer in this post are the following:

1. What does it mean for an integer to be divisible by another integer?
2. Is zero divisible by every integer?
3. Are there any integers that are divisible by zero?

Note that this is a pretty technical article with some fairly sophisticated mathematics. You should only read this post if you are genuinely interested in why division by zero is impossible.

So take a deep breath, and let’s begin…

We say that 42 is divisible by 7 because 42 = 7·6. The number “6” itself isn’t particularly important here, but what is important is that 6 is an integer. In other words, 42 is divisible by 7 because there is an integer k such that 42 = 7k.

In general, an integer n is divisible by an integer d if there is another integer k such that n = dk.

In practice we can check if n is divisible by d simply by dividing n by d in our calculator (or sometimes by using the divisibility tricks I mentioned earlier). If the answer is an integer, then n is divisible by d. If the answer is not an integer (it contains digits after the decimal point), then n is not divisible by d.

For example, when we divide 42 by 7 we get 6, an integer. So 42 is divisible by 7.

As another example, every integer n is divisible by 1. Indeed, n = 1·n, and n is an integer.

What about the integer 0? Is 0 divisible by 7?

Yes it is because 0 = 7·0, and 0 is an integer.

In fact, if m is any nonzero integer, then 0 is divisible by m. Indeed, 0 = m·0.

This fact is worth emphasizing since students often get confused by it.

Zero is divisible by every nonzero integer!

Note in the bold statement above the word “nonzero.” Isn’t 0 also divisible by 0 according to that definition? After all, if we set m = 0 in the equation “0 = m·0,” we then get 0 = 0·0 which is a true statement! We will talk about this more in just a bit.

Let’s now check if there are any integers n which are divisible by 0. If n is divisible by 0, then there is an integer k such that n = 0·k. But 0·k = 0. So in other words, if n is divisible by 0, it follows that n must be equal to 0.

In other words, there are no nonzero integers that are divisible by 0!

Note that we have not shown that 0 is divisible by 0. We only proved the conditional statement “if an integer is divisible by 0, then that integer must be 0.” We still need to check if 0 actually is divisible by 0.

So let’s assume for a moment that 0 is divisible by 0. This means that there is an integer k such that 0 = 0·k. But every single integer satisfies this equation. Just to pick two specific values, 0 and 1 both satisfy this equation. This means that 0/0 = 0 and 0/0 = 1. It follows that 0 = 1. But 0 and 1 are different integers. It follows that 0 cannot be divisible by 0. Cool, huh?

Maybe your head is spinning a little after this, so take a deep breath, take some time away from this post and then come back to re-read it – if you are interested in why we can’t divide by zero!

As mentioned, this info is not relevant for SAT math, but (for me at least) it is very interesting and can help raise your level of mathematical maturity. The question of why division by zero is not possible is one of the big holes students have in their understanding, so I hope that after reading this post you are now a little wiser!

Take care! I’ll be back with more SAT math relevant material next week…

Hi all,

This is just a short message to let you know that parts of SATPrepGet800 website will be down at times throughout September.

We are actually updating the site so it looks more modern and is easier to navigate.

So I’ll keep you updated on the progress.

All the best!

A while back I created a thread that explained how SAT math problems involving remainders can be solved quickly and easily. Quickly take a look at these posts to refresh your memories on what I wrote. Click on these links for part 1 (calculator algorithms) , 2 (cyclical nature of remainders) and 3 (recognizing remainder problems) for the posts in this thread.

I realized that I can share with you certain ‘tricks’ that you can employ to quickly and easily divide numbers without using long division or a calculator. Some of these tricks will actually take less time than typing the numbers into your calculator. With practice these tricks can become second nature to you which of course means that you can save precious time on the day of the SAT.

Some of these tricks will be obvious to you, but that is only because you have practiced the trick for that particular type of number so often that it has already become second nature to you.

Here are some easy tricks that you may already know:

• An integer is divisible by 2 precisely when the last digit is 0, 2, 4, 6 or 8.

For example, 326 is divisible by 2, whereas 327 is not.

• An integer is divisible by 3 precisely when the sum of its digits is divisible by 3.

For example 477 is divisible by 3 because 4 + 7 + 7 = 18, and 18 is divisible by 3.

What about 5215? Well, 5 + 2 + 1 + 5 = 13. Since 13 is not divisible by 3, neither is 5215

• An integer is divisible by 4 precisely when the number formed by taking just the last two digits of the integer is divisible by 4.

For example, 2,136,416 is divisible by 4 simply because 16 is divisible by 4.

What about 532,211. Well 11 is not divisible by 4. So neither is 532,211.

• An integer is divisible by 5 precisely when the last digit is 0 or 5.

So 6,235 is divisible by 5, but 2,112,321 is not divisible by 5.

• An integer is divisible by 6 if it is divisible by 2 and by 3.

Let’s check if 2,132,478 is divisible by 6. Well this number ends in 8, so it is divisible by 2. Also, 2 + 1 + 3 + 2 + 4 + 7 + 8 = 27. Since 27 is divisible by 3, so is 2,132,478. Since this number is divisible by both 2 and 3, it is divisible by 6.

• An integer is divisible by 9 precisely when the sum of its digits is divisible by 9.

For example, 477 is divisible by 9 because 4 + 7 + 7 = 18, and 18 is divisible by 9.

What about 5214? Well, 5 + 2 + 1 + 4 = 12. Since 12 is not divisible by 9, neither is 5214. (Note however, that 5214 is divisible by 3).

• An integer is divisible by 10 if it ends in a 0.

So 5960 is divisible by 10, and 7655 is not.

Here are a few more – these are advanced divisibility tricks:

Please take note that these last few tricks are not standard at all, and almost nobody knows them. I am including them more for fun, and because it makes the post more complete (otherwise the only numbers “left out” are 7 and 8). But most students can skip them.

• Here is a nice little algorithm for testing if an integer is divisible by 7: Double the last digit of the integer and subtract this value from the original integer with the last digit removed. If this new number is divisible by 7, so is the original number. Otherwise it is not.

Let’s check if 5362 is divisible by 7:

536 – 2(2) = 532

53 – 2(2) = 49

Since 49 is divisible by 7, so is 532. Since 532 is divisible by 7, so is 5362.

• An integer is divisible by 8 precisely when the number formed by taking just the last three digits of the integer is divisible by 8.

For example, let’s check if 2,136,416 is divisible by 8. Well when we divide 416 by 8 we get 54. So 416 is divisible by 8, and therefore so is 2,136,416.

• Is there a way to test for divisibility by 11? Yes there is. Add up every other digit beginning with the second digit from the left, and then subtract the remaining digits. If this new number is divisible by 11, so is the original number. Otherwise it is not.

Let’s check if 28,765 is divisible by 11. Well (8 + 6) – (2 + 7 + 5) = 0. Since 0 is divisible by 11, so is 28,765.

As mentioned, I do not recommend worrying about these last few for the SAT. I just included them for those of you that might be interested in learning some more advanced tricks. Note that there are many, many more advanced divisibility tricks out there. If I were to include them all, this post would be ten pages long, so I needed to stop here. But feel free to post your favorites in the comments and we can discuss them. In any case, with practice you will be using divisibility tricks without even realizing it!

Here is a video containing this information:

 

You can practice using problems found in my books.

If you have any questions or comments let me know… If you found the post helpful, why not use the share button below to let your friends know of this post too? A share would be much appreciated.

Speak next week!

Combinations On The SAT

Continuing on from last week, today I would like to continue discussing more advanced methods for solving counting problems.

Two weeks ago we discussed some more basic methods for solving counting problems on the SAT. These methods can be viewed by clicking the following link: Listing and the Counting Principle

And last week we talked about permutations. This article can be viewed here: Permutations 

Today I want to focus on another more advanced counting techniques – Combinations.

Combinations

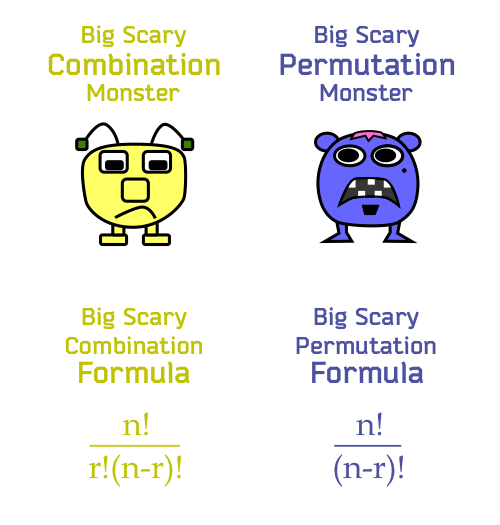
First a definition: A combination is just a subset containing a specific number of the elements of a particular set. The number of combinations of n things taken r at a time is

For example, the number of combinations of {1, 2, 3} taken 2 at a time is

These combinations are 12, 13, and 23.

Note that 21 is the same combination as 12 (but 12 and 21 are different permutations). More on this below…

Just like the permutation formula, you do not need to know the combination formula for the SAT. You can do this computation very quickly on your TI-84 graphing calculator. To compute ₃C₂, type 3 into your calculator, then in the Math menu scroll over to Prb and select nCr (or press 3). Then type 2 and press Enter. You will get an answer of 3.

Difference Between Permutations And Combinations

Let me quickly tell you the difference between permutations and combinations: “Permutation” is just a fancy word for arrangement. Whenever you are counting the number of ways to arrange things you can use the permutation formula that I shared with you last week (or better yet the nPr feature in your calculator). So if you want to “order” things, “stack” things, “rearrange” things, choose specific tasks for people, etc, you would use permutations.

Example 2:

Here is our first SAT math problem where combinations can be used:

2. A chemist is testing 5 different liquids. For each test, the chemist chooses 3 of the liquids and mixes them together. What is the least number of tests that must be done so that every possible combination of liquids is tested?

First note that we can solve this problem by simply listing all of the possibilities. But be careful! This is just a bit tricky.

In the following list a * means we are choosing that liquid, and an O means we are not:

***OO      *OO**
**O*O      O***O
**OO*      O**O*
*O**O      O*O**
*O*O*      OO***

We see that there are 10 combinations.

Alternatively, we can obtain the solution quicker by using what we have learned about combinations: We are counting the number of ways to choose 3 of the 5 liquids. This is ₅C₃ = 10.

Example 3: 

And here is a more difficult SAT math problem that can be solved quickly using combinations:

3. Any 2 points determine a line. If there are 18 points in a plane, no 3 of which lie on the same line, how many lines are determined by pairs of these 18 points?

We need to count the number of ways to choose 2 points from 18. This is the combination ₁₈C₂ = 153.

Let me end this post by saying that on the SAT I have not seen a problem where you absolutely NEED to use permutations or combinations. You can always do counting problems on the SAT either by forming a careful list, or by using the counting principle. But every now and then using permutations or combinations will get you the answer much more quickly.

And that’s all you need to know about counting problems for the SAT, but if you want more practice problems I suggest you take a look at my 28 SAT Math Lessons Series. Click on the picture below for more information about these books.

If you liked this article, please share it with your Facebook friends:

And if you have any questions on this topic, then please post them on my Facebook wall:

Permutations On The SAT

Today I would like to talk about some more advanced methods for solving Counting Problems. The content presented here and in next week’s post is meant for more advanced students whose current SAT math score is at least a 600.

Last week we discussed some more basic methods which can be viewed by clicking the following link: Listing and the Counting Principle.

Please quickly review last week’s post before going on to the content below. Five minutes of review will really solidify the concepts from that post and will make the information in this post easier to learn.

Factorials

First let’s discuss factorials. The factorial of a positive integer n, written n!, is the product of all positive integers less than or equal to n.

n! = 1·2·3…n

0! is defined to be 1, so that n! is defined for all nonnegative integers n.

The definition for 0! may seem strange to many of you. Mathematicians have made this definition so that the formula n! = n(n – 1)! will be true for all positive integers n. Note that with this definition 1! = 1·0!

Also, I could make jokes about being really excited about factorials because we use an exclamation point in the definition – but let’s leave these for another day…

Permutations

Now a permutation is just an arrangement of elements from a set. The number of permutations of n things taken r at a time is

For example, the number of permutations of {1, 2, 3} taken 2 at a time is

These permutations are 12, 21, 13, 31, 23, and 32.

The good news is that on the SAT you do not need to know the permutation formula. You can do this computation very quickly on your graphing calculator. To compute ₃P₂, type 3 into your calculator, then in the Math menu scroll over to Prb and select nPr (or press 2). Then type 2 and press Enter. You will get an answer of 6.

As always, let’s internalize these principles by putting them into practice. Here is a simple SAT problem that can be solved using permutations.

Examples

1. Four different books are to be stacked in a pile. In how many different orders can the books be placed on the stack?

Okay, now you could imagine stacking the books in different ways. Let’s try this by listing the different permutations. To distinguish the books let’s make one red, one blue, one yellow, and one green. Let’s abbreviate each book’s color by using its first letter.

rbyg rbgy rybg rygb rgby rgyb
bryg brgy byrg bygr bgry bgyr
yrbg yrgb ybrg ybgr ygrb ygbr
grby gryb gbry gbyr gyrb gybr

We now see that there are 24 arrangements.

The thing is that on the actual SAT I would rather not take the time to write out each permutation. There is a chance, under the time pressure of the test, that an error could be made or too much time could be spent checking that I have not duplicated any of the permutations.

Here are two more solutions that are much more efficient.

First we can use the counting principle that we reviewed in last week’s post: There are 4 possible books for the bottom of the stack. After placing the first book, there are 3 possible books that can go on top of the bottom book, then 2 books for the next position, and then 1 book for the top of the stack. Using the counting principle we get a solution of (4)(3)(2)(1) = 24 arrangements.

Or we can solve this problem even faster by using our new knowledge of permutations: There are 4 books, and we are arranging all 4 of them. Therefore there are ₄P₄ = 4! = (1)(2)(3)(4) = 24 arrangements.

I think you would agree that the last solution is much faster than the original solution by listing.

Let’s do one more SAT problem:

2. Three light bulbs are placed into three different lamps. How many different arrangements are possible for three light bulbs of different colors – one red, one green, and one yellow?

Again, we can list all the possibilities, abbreviating each color by using the first letter.

rgy ryg gry gyr yrg ygr

We can easily see that there are 6 arrangements.

And again, we can also use the counting principle: There are 3 possible lamps to place the red bulb in. After placing the red bulb, there are 2 lamps to place the green bulb in. Finally, there is 1 lamp left to place the yellow bulb in. By the counting principle we get (3)(2)(1) = 6 arrangements.

And finally we can solve this problem very quickly using our knowledge of permutations: There are 3 light bulbs, and we are arranging all 3 of them. So the number of arrangements is ₃P₃ = 3! = 1·2·3 = 6.

There are many more of these types of problems in my 28 SAT Math Lessons Series. Click on the picture below for more information about these books.

If you liked this article, please share it with your Facebook friends:

And if you have any questions on this topic, then please post them on my Facebook wall:

Click the following link to learn even more advanced counting techniques: Combinations

Counting Problems

This week I would like to start a thread on ‘counting.” Although counting sounds like it should be an easy concept, students tend to find SAT math problems that involve counting among the most difficult.

Writing A List

Okay let’s start with the most basic strategy, namely ‘writing a list’… Sometimes the easiest way to count the number of possibilities is to simply list them all. When doing this it is important that you have a systematic way of forming your list. This will reduce the likelihood of missing something, or listing something twice.

With that said, let’s look at a simple SAT counting problem:

1. A menu lists 2 meals and 3 drinks. How many different meal drink combinations are possible from this menu?

Some of you may already be able to solve this problem very quickly, but let’s first solve this by writing a list..

Let’s make up 2 meals and 3 drinks, say chicken and fish for the meals, and water, soda, and juice for the drinks. Now let’s list all meal-drink combinations. To save time we will abbreviate each meal and drink by using its first letter.

cw  cs  cj  fw  fs  fj

We see that there are 6 meal-drink combinations.

Now, as many of you probably already realize, we can actually solve this problem very quickly by using the counting principle.

(2)(3) = 6

The Counting Principle

The counting principle says that if one event is followed by a second independent event, the number of possibilities is multiplied. The 2 events in the above problem are “choosing a meal,” and “choosing a drink.”

Now let’s get a little more advanced and look at the counting principle in full generality.

More generally, the counting principle says that if E₁, E₂, …, E_n are n independent events with m₁, m₂, …, m_n possibilities, respectively, then event E₁ followed by event E₂, followed by event E₃, …, followed by event E_n has  m₁∙m₂ ∙∙∙ m_n possibilities.

My head is spinning after looking at all these E’s and m’s, so let’s try a problem to see this principle in action…

2. How many integers between 9 and 300 have the tens digit equal to 2, 3, or 4 and the units digit (ones digit) equal to 5 or 6?

Let’s first solve this using the more general version of the counting principle:

There are 2 possibilities for the ones digit (5 or 6). There are 3 possibilities for the tens digit (2, 3, or 4). There are 3 possibilities for the hundreds digit (0, 1, or 2). The counting principle says that we multiply the possibilities to get (2)(3)(3) = 18.

That’s not so bad, is it?

Note that we could also solve this problem by writing a list:

Let’s simply list all the numbers in increasing order:

25  26  35  36  45  46  125  126  135  136  145  146  225  226  235  236  245  246

And that’s it. Once again we see that the answer is 18.

A Difficult SAT Math Counting Problem

Okay, let’s do one more SAT counting problem. This one is Level 5:

3. How many integers between 3000 and 4000 have digits that are all different and that increase from left to right?

The best way to solve this is by writing a list:

As you can see, there are 20 integers in this list. It would be good to take note that we only wrote down the necessary information when forming our list. For example, the second entry was just written “7” instead of “3457.” This will save a substantial amount of time.

Also a clear and definite pattern was used in forming this list. In this case the list was written in increasing order. This will minimize the risk of duplicating or leaving out entries.

Follow the advice given here with  similar problems you see on the SAT and you will be able to answer them quickly and efficiently.

For more advanced counting techniques take a look at my articles on Permutations and Combinations.

Where To Find More Practice Problems

You can find lots of SAT math problems to practice with in my 28 SAT Math Lessons Series. Each of these books contains hundreds of problems, most with several different solutions. Click on the picture below for more information about these books.

If you liked this article, please share it with your Facebook friends:

And if you have any questions on this topic, then please post them on my Facebook wall:

Speak to you soon!

Before I get into the bulk of this message I would like to first mention  that this offer is available for only 48 hours. So you really have to act now if you want to take advantage of this offer.

Now let me explain how this offer would be good for your SAT prep…

If you are using the College Board’s Blue Book, then you are probably aware that the College Board’s answers to their own SAT math problems are not very efficient.

The issue that teachers, tutors and students have with the College Board’s solutions is that they are very tedious in their approach to answering the question – they use long methods that you would expect a fresh-out-of-college school math teacher to use to solve problems. These methods are not time friendly. On the SAT you would be eating up valuable time using these methods.

Of course, it would be wiser to use more effective and efficient methods to solve SAT problems. Answering problems quickly will give you time to recheck your answers (preferably by using a different method) to ensure that you have gotten the correct answer.

There is now a simple solution – my latest book release: SAT Prep Official Study Guide Math Companion. The good news is that Amazon currently has this book on sale for only $9.49. My other books normally retail for $29.99 on Amazon – so this is a great savings.

This book has all the solutions to all the SAT math tests in the Blue Book – that’s tests 1 through 10, and the DVD test.

Please note that this sale is only guaranteed for the next 48 hours. The price will be going back up to $29.99 after that!

There are several reasons to use my solutions instead of the College Board’s. First, my solutions are extremely efficient. They will save you an enormous amount of time during the exam. You will be able to fly through the test and have enough time to spare to re-check your answers. Second, by reviewing and internalizing my solution methods you will become proficient in all of the most important SAT math strategies for raising your SAT score in a quick, efficient manner. Note that all relevant mathematical concepts and definitions are naturally reviewed as they come up in the questions and solutions of actual SAT math problems. And third, I do not include just one method of solution for each question – problems often have multiple efficient solutions to the problems for you to solve in different ways.

The benefit of having multiple solutions is that you can pick and choose which method is best for you to solve SAT problems on test day. Also, learning multiple solutions for SAT math problems will allow you to “re-check” answers you have gotten on test day by solving each problem with a different method. This will ensure that you are indeed answering the problems correctly.

So take a look at, what I think is, the best SAT prep book available now. For only $9.49 from Amazon, you can’t go wrong!

You only have 48 hours left, so act now!

Hey all, how are you today?

If you remember, last week we discussed the triangle rule. I provided a few SAT problems where the triangle rule was extremely useful, and it was not hard to see how this simple rule should be applied.

Recall that the triangle rule states that that the length of the third side of a triangle is between the sum and difference of the lengths of the other two sides.

This can be written symbolically as “difference < x < sum” where x is the third side of the triangle.

Today let us look at another type of problem that the triangle rule should be used to solve.

Problems involving distances between three points can often be solved using the triangle rule. After all, when you plot three points and look at the distances between each pair of points, you are looking at the lengths of the sides of a triangle.

Try plotting three points on a piece of paper and you you will immediately see what I mean.

There is a small catch however – sometimes when you plot three points, the points can be collinear: that is they all lie on the same line.

This means that the symbol “<” in the triangle rule should be replaced by the symbol “≤.”

In other words, this time we write “difference ≤ x ≤ sum.”

Let’s look at an example that could appear on the SAT.

Points Q, R and S lie in a plane. If the distance between Q and R is 18 and the distance between R and S is 11, which of the following could be the distance between Q and S?

 

Okay, to solve this problem, of course we are going to use the triangle rule… In this case, if Q, R and S form a triangle, then the length of QS is between 18 – 11 = 7 and also 18 + 11 = 29. The extreme cases 7 and 29 form straight lines. In this problem that is fine, so the distance between Q and S is between 7 and 29, inclusive. Thus, the answer is choice (E).

You can see how quickly this problem can be solved when the triangle rule is used.

If you want more basic info on the triangle rules, them please see my previous post. There are lots of problems with solutions that you an sink your teeth into.

Until next time…

This week, let’s get back to some mathematical content. Today I would like to discuss the triangle rule.

I consider using the triangle rule to be an advanced SAT prep strategy. It comes up on level 4 and 5 problems on the SAT and the only reason so many students get these problems wrong is because they have never been taught the rule in school.

The triangle rule states that that the length of the third side of a triangle is between the sum and difference of the lengths of the other two sides.

So, for example, if we knew that a triangle had two sides of lengths 5 and 7, respectively, then we could find the possible lengths of the third side by using the triangle rule. Simply note that the sum is 7 + 5 = 12 and the difference is 7 – 5 = 2. Therefore the the length of third side lies between 2 and 12.

Let’s get our heads around this with another short example: If a triangle has sides of lengths 2, 5, and x, then we have that 5 – 2 < x < 5 + 2. That is, 3 < x < 7.

The triangle rule is a very easy concept to understand. Again, the only reason why these are considered tough problems is because the rule is not emphasized in school, and sometimes not taught at all.

So let’s really get this rule into practice by solving some problems that might actually appear on the SAT.

The lengths of the sides of a triangle are x, 8, and 15, where x is the shortest side. If the triangle is not isosceles, what is a possible value of x?

The triangle rule tells us that 15 – 8 < x < 15 + 8. That is, 7 < x < 23. Since x is the shortest side, x < 8. So we must choose a number between 7 and 8. If this were a grid in problem, we could grid in 7.1 or any other decimal or improper fraction between 7 and 8. But inputting 7 or 8 as the answer would be incorrect as these integers are not between 7 and 8!

Okay, one more. I have included a few more examples in this post than I normally do. The solutions to these problems are so quick and easy that there is no point padding them out with unnecessary fluff – I would much rather provide examples of problems that require the rule to be used. This one is a multiple choice question.

If x is an integer greater than 5, how many different triangles are there with sides of length 3, 5 and x?

 

The triangle rule tells us that 5 – 3 < x < 5 + 3. That is, 2 < x < 8. Since x is an integer greater than 5, x can be 6 or 7. So there are two possibilities, choice (B).

My students find these problems very straight forward. I hope you do too. But it can be easy to forget the rule you need to use if you do not practice enough with it. If you want more practice, then please see the triangle rule SAT math problems in my books. These have more examples of problems that need to use this rule for efficient and correct answering.

Last week I gave you a diagram that guides you through the 7 steps of efficient SAT math prepping.

This diagram referenced my material within the various steps. However, some students that read this blog mentioned to me that they would like to use alternative prep materials with the 7 steps. I understand that not everyone wants to use some or all of my books to prep – for example, you may already have alternative quality resources – so I have created a slightly different diagram for you to follow.

This diagram below does not reference my books so you can use any other material you have to efficiently prepare for the SAT. Simply follow the stages and you will get to your target score within 4 months of studying for just 10 – 20 minutes per day. Of course, you will need to set aside more time on days that you are taking a timed test from the College Board’s Blue Book (approximately once per month).

Note that everyone preparing for the SAT should have the Blue Book regardless of what other preparation is undertaken.

Make sure that you continually retry questions that you have gotten wrong until you can get each question correct on your own. This includes any questions you have gotten wrong while taking practice tests from the Blue Book. Always wait at least a few days between attempts.

Other than that, just follow the diagram and you should be on the path to your SAT math target score.