500 New SAT Math Problems
Just 19.99 on Amazon

Hi everyone! The latest edition of 500 New SAT Math Problems is now available in paperback from Amazon. This edition just has been modified from the previous edition to account for the changes on the Digital SAT.

The paperback is now on sale on Amazon for only $19.99. Note that once the sale ends (by the end of today), the price of this book will go up to $42.99. 

The promotion has ended. Thanks to everyone who participated. The book is now available at its regular price here: 500 New SAT Math Problems

If you have any questions, feel free to contact me at steve@SATPrepGet800.com 

Thank you all for your continued support!

A Trick For Free Two Day Shipping

I would like to finish this post with a little trick you can use to get free 2 day shipping on any of the books you decide to purchase without making any additional purchases. If you have never used Amazon Prime you can sign up for a free month using the following link.

Sign Up For Amazon Prime For Free

If you have already had a free trial of Amazon Prime you can simply open up a new Amazon account to get a new free trial. It just takes a few minutes! You will need to use a different email address than the one you usually use.

This next part is very important! After you finish your transaction, go to your Account, select “Manage my prime membership,” and turn off the recurring billing. This way in a month’s time Amazon will not start charging you for the service.

After shutting off the recurring billing you will still continue to receive the benefit of free 2 day shipping for one month. This means that as long as you use this new Amazon account for your purchases you can do all of your shopping on Amazon for the next month without having to worry about placing minimum orders to get free shipping.

Just be aware that certain products from outside sellers do not always qualify for free shipping, so please always check over your bill carefully before you check out.

Well I hope you decide to take advantage of this very special offer, or at the very least I hope you will benefit from my Amazon “free 2 day shipping trick.” Here is the link one more time:

Sign Up For Amazon Prime For Free

If you think your friends might be interested in this special offer, please share it with them on Facebook:

Thank you all for your continued support!

A few weeks ago I wrote a blog post on how my various Get 800 SAT prep books should be used in conjunction with the College Board’s Official SAT Study Guide.

I have had a few questions about the process and I thought that it might be better to explain the 7 steps in a visual format. So I created a flow chart that takes you through the preparation process that I recommend for SAT math.

The diagram below does not tell you which step is which. Instead it takes you through each stage while asking questions that may restart the process to an earlier stage. This chart is easier to follow than the text on my website or on the previous blog post.

Also, although this flow chart specifically names books to use for your math prep, you could, in fact, replace the names of my books with alternate SAT prep material.

So for example, Instead of using the 28 SAT Math Lessons Beginner, Intermediate or Advanced books, you could essentially replace these books with level 1 – 3, 2 – 4 or 3 – 5 SAT math problems respectively.

Instead of using my 320 SAT Math Problems book to focus on areas you are not strong in, you could instead find the kinds of problems you need help with from other sources.

At the end of the flow chart is an instruction that says learn the strategies from The 32 Most Effective SAT Math Strategies. Again this can be replaced by other sources at your disposal that explain math strategies.

Finally, after taking a College Board Blue Book test, I advise that you use my Companion to compare solutions, as I believe the College Board’s solutions are not the best to use on test day and mine are more time efficient. Again, if you have alternate solutions from other sources feel free to use these instead of mine.

I may make another flow chart that is generic in terms of what products to use but the process will be the same no matter who is preparing for SAT math. I simply name my own products in this diagram because they fit perfectly within the efficient SAT math prep process that I recommend.

By now, you may have heard that College Board is going to redesign the SAT.

Before everyone begins to panic, you should be aware that the new test will not be administered in the near future. So if you are preparing to take the SAT within the next 4 months (which is the amount of time I generally recommend for SAT preparation) then this change will not affect you. So there is no need to worry.

In February, 2013  The College Board announced that the SAT will be changed. The new test will “strongly focus on the core knowledge and skills that evidence shows are the most important to prepare students for the rigors of college and career” as quoted by Peter Kaufman, the Vice President of the College Board.

Interestingly, the last time the College Board announced a redesign in 2004, the actual test was not introduced to students until 2005. In fact, it was the PSAT that changed first. This gave students an indication of the changes that would be introduced to the SAT.

So even though the College Board has not stated this (or any details for that matter) it seems to me that we should look out for a new style PSAT in 6 months to a year – early to mid 2014 – and expect a new SAT to come out about 6 months after that time.

So why the change now? Well the SAT has never been a test that remained static. It has been developed and changed with the input of Colleges, Universities and High Schools in order to better measure the test takers’ ability. For example, in 2005 there was a change to assess writing skills, and in fact, before that, the math and verbal sections used to be just one section.

So for now, there is no need to worry. But I will keep you updated as more news is released on the proposed SAT redesign.

It now seems that I have many SAT math prep books, even to me. So I would like to make clear why and when you would use each of my books.

I would first like to state that there is no duplicate material in any of my books. Each of my books have different SAT problems, and each title is designed for a specific reason. So with this in mind, let’s see how effective test preparation can be achieved using my books together with The College Board’s Blue Book.

So how should you first begin your prep? Well, you should gauge your score by taking a practice test from the College Board’s Official SAT Study Guide (the Blue Book). You should take this practice SAT under timed conditions. Use the Blue Book to tell you your current SAT score. It does not matter how many questions you got wrong, and you do not need to worry about reviewing these questions at this stage – we just want to know your score without any preparation. If you have recently taken an SAT or PSAT, you can use the score from that test in place of taking a test from the Blue Book.

Now it is time to begin your actual SAT math prep. You have a score and now you can use the appropriate level of “28 SAT Math Lessons to Improve Your Score in One Month.” These books will easily guide you to a higher score. All you have to do is complete one lesson per day, and after 28 days you should see a significant jump in your score level. My students like these books because they take out all the guess work out of what to study. These books will hand hold you to a higher SAT score.

I have three books in this series that can be used as standalone guides. So if your score is below 500 use the Beginner Course. If your score is between 500 and 600 use the Intermediate Course. If your score is over 600, use the Advanced Course unless you see that you are getting several Level 1, 2, or 3 questions wrong, Then you should begin with the Intermediate Course.

After completing the appropriate 28 Lessons book it is time to take another SAT from the College Board’s Official Study Guide under timed conditions to get your new math score.

Again, you will probably get several problems incorrect, but this time you should go over your mistakes carefully. “The Complete Official SAT Study Guide Companion“ can be used to compare your solutions to mine. You should certainly review all of your incorrect solutions, but feel free to look at solutions to problems you got correct just to compare your solutions to mine. This book provides many different ways to solve each test problem in the College Board’s Blue Book.

You may be tempted to review the problem you got wrong in the Blue Book by looking at the College Board’s solutions. This is a mistake. Although all of their solutions are correct, they are mostly tedious and inefficient. The College Board has no interest in providing students with time-saving strategies and foolproof tactics for ensuring correct answers. They only provide straightforward solutions that could actually be quite harmful to your SAT math preparation. This is why you would use “The Complete Official SAT Study Guide Companion”.

If you are happy with your new SAT math score, then you can go ahead and take your SAT. If you still want to score higher, it’s time to wash, rise and repeat:

If your score has gone to the next score range, then you can begin using the next “28 SAT Math Lessons” book in the series. For example, if your previous score was a 450, and your new score is a 550, you can now move up from the Beginner Course to the Intermediate course.

But if your score is still in the same range (score has increased since using 28 SAT Math Lessons, but it is still within the same ‘level’), you should use “320 SAT Math Problems Arranged by Topic and Difficulty Level“ to get past your sticking points. For example, if your previous score was a 300, and your new score is a 400, then you probably still need practice with Level 1 and 2 problems. Practice these problems from the 320 Problems book for a few more weeks, then take another SAT from the College Board’s Official Study Guide under timed conditions to get your new math score. Again use “The Complete Official Study Guide Companion” to compare your solutions to mine.

The nice thing about “320 SAT Math Problems Arranged by Topic and Difficulty Level” is that you can flick to the page with the types of problems that are giving you trouble. So if you are having trouble with Level 2 geometry, you can work on those problem types alone and master that particular level of that subject.

If you have completed the Advanced 28 Lessons book and would like additional practice, use “320 SAT Math Problems Arranged by Topic and Difficulty Level”  and focus on the Level 4 and 5 problems from this book.

Finally, for real SAT math mastery – especially if you are aiming for a perfect or near perfect score of 800, read “The 32 Most Effective SAT Math Strategies“ to review each of my strategies one by one and practice applying them to a wide range of SAT Math Problems. My strategies consist of quick and efficient ways to solve seemingly difficult SAT math problems with real ease.

So take another practice test and hopefully you will be at a score level you can be very happy with  – and the colleges you are applying to will be happy with your score as well – it’s time to take the SAT for real…

Hi everyone. How are we doing today?

This week I would like to help you solve SAT math problems that involve finding the greatest common divisor and least common multiple of a set of positive integers. But before I even get to this week’s post, I would like to go back to last week’s post on Prime Factorizations where I asked you to draw a factor tree for the number 6137. You can find that post here: Integers, Prime Numbers, and Prime Factorizations

Solution to Last Week’s Question

Let’s compare our drawings.

As I mentioned last week, it’s not artistic merit I am looking for, but rather that the numbers in your ‘tree’ are correct.

Let’s start by taking the square root of 6137. Punching that into your calculator (TI-84 or equivalent) we see that we get about 78.3. So using the tip that I gave to you last week, we will divide 6137 by the prime numbers less than 78.3.

The primes less than 78.3 are 2, 3, 5, 7, 11, 13, 17, 19, 23, 29, 31, 37, 41, 43, 47, 53, 59, 61, 67, 71 and 73.

The good news is that we do not need to divide by all of these numbers to get to find our factors – let me explain: Dividing by 2, 3, 5, 7, 11, and 13 do not produce integers, but 6137/17 = 361.

Now, the square root of 361 is exactly 19 (this is easily checked in your calculator). So 361 = (19)(19) = 19².

So 6137 = 17 · 19².

Here is my factor tree:

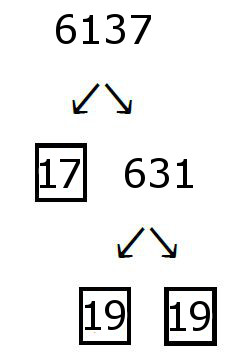
How does your tree look compared to mine? Get in touch if you have any questions regarding this…

GCD and LCM

Now for the main part of the post… The greatest common divisor (gcd) of a set of positive integers is the largest positive integer that each integer in the set is divisible by. The least common multiple (lcm) of a set of positive integers is the smallest positive integer that is divisible by each integer in the set.

Example 1:

Let’s find the gcd and lcm of 9 and 15.

There are a few ways we can do this.

First method: The factors of 9 are 1, 3 and 9. The factors of 15 are 1, 3, 5 and 15. So the common factors of 9 and 15 are 1 and 3. Therefore we see that gcd (9,15) = 3.

The multiples of 9 are 9, 18, 27, 36, 45, 54, 63,… and the multiples of 15 are 15, 30, 45,.. We can stop at 45 because 45 is also a multiple of 9. Therefore we see that lcm (9,15) = 45.

Second method: We first find the prime factorizations of 9 and 15. We see that 9 = 3² and 15 = 3 · 5. To find the gcd we multiply together the smallest powers of each prime from both factorizations, and for the lcm we multiply the highest powers of each prime. So we have gcd (9,15) = 3 and we have lcm (9,15) = 3² · 5 = 45.

Now take note – If you have trouble seeing where the gcd and lcm are coming from here, it may be helpful to insert the “missing” primes. In this case, 5 is missing from the factorization of 9. So it might help you if you write 9 = 3² · 5º. Now we can think of the gcd as 3¹ · 5º = 3.

Third method: Your calculator can actually do this for you! On your TI-84 calculator press MATH, scroll right to NUM. For the gcd press 9, type 9,15 and press ENTER. You will see an output of 3. For the lcm press 8, type 9,15 and press ENTER for an output of 45.

Example 2:

Let’s try another example: Find the gcd and lcm of 100 and 270.

The prime factorizations of 100 and 270 are 100 = 2² · 5² and 270 = 2 · 3³ · 5. So gcd (100,270) = 2 · 5 = 10 and lcm (100,270) = 2² · 3³ · 5² = 2700.

If we were to insert the ‘missing’ primes in the prime factorization of 100 we would get 100 = 2² · 3º · 5². So we can think of the gcd as 2¹ · 3º · 5¹ = 10.

Example 3:

Okay, now let’s try an SAT math question…

What is the least positive integer divisible by the integers 3, 7 and 14?

Calculator method: The question is actually asking for the least common multiple of 3, 7 and 14. Your calculator can only do two at a time. So first compute lcm (3,7) = 21, and then compute lcm (21,14) = 42, choice (D).

Simple!

Solution by Starting with choice (E): Begin by looking at choice (E) since it is the smallest. 28/3 comes to approximately 9.33 in our calculator. Since this is not an integer, 28 is not divisible by 3. We can therefore eliminate choice (E). We next try choice (D).

42/3 = 14, 42/7 = 6, 42/14 = 3

Since these are all integers, the answer is choice (D).

For more information on this strategy see the following blog post: Starting With Choice (C) – A Basic SAT Math Strategy.

Example 4:

Now here is a much more difficult example for us to try together:

The integer k is equal to m² for some integer m. If k is divisible by 6 and 40, what is the smallest possible positive value of k?

Did you realize that we are looking for the smallest perfect square that is divisible by the least common multiple of 6 and 40? Let’s find some prime factorizations: 6 = 2 · 3, and 40 = 2³ ·5. So lcm (6,40) = 2³ · 3 · 5. The least perfect square divisible by this number is 2⁴ · 3² · 5² = 3600.

It’s not too hard when you know how to compute the lcm.

This was quite a long blog post, but I hope you have received some good value from it.

If you liked this article, please share it with your Facebook friends:

And if you have any questions on this topic, then please post them on my Facebook wall:

I will answer your questions right away.

Speak to you soon!

Hey… How are you doing?

I would like to begin today with a few simple definitions of terms that appear in number theory problems on the SAT. All too often I have students attempting SAT math problems from the College Board Blue Book without even knowing what some of these terms mean. So here are a few essential definitions that you should make sure you are very familiar with.

Definitions:

The integers are the counting numbers together with their negatives.

…,-4, -3, -2, -1, 0, 1, 2, 3, 4,…

The positive integers consist of the positive numbers from the above list.

1, 2, 3, 4,…

Next we have prime numbers.

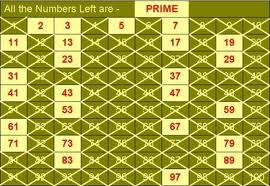
A prime number is a positive integer that has exactly two factors (1 and itself). Here is a list of the first few primes:

2, 3, 5, 7, 11, 13, 17, 19, 23,…

Note that 1 is not prime. It only has one factor.

A little trick:

Here is a quick trick for determining if a large number is prime: take the square root of the integer and check if the integer is divisible by each prime up to this square root. If not, the number is prime.

For example let’s try to figure out if 3001 is a prime number. Note that when we take the square root of 3001 in our calculator we get approximately 54.8. Now with our calculators we divide 3001 by 2, 3, 5, 7, 11, 13, 17, 19, 23, 29, 31, 37, 41, 43, 47, and 53 (all the prime numbers below 54.8). Since none of these are integers, 3001 is prime.

The Fundamental Theorem of Arithmetic

The fundamental theorem of arithmetic says “every integer greater than 1 can be written “uniquely” as a product of primes.”

The word “uniquely” is written in quotes because prime factorizations are only unique if we agree to write the primes in increasing order.

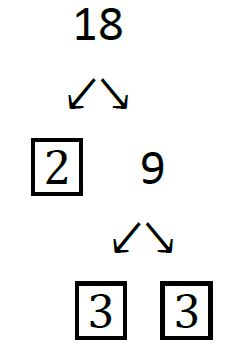
For example, 6 can be written as 2 · 3 or as 3 · 2. But these two factorizations are the same except that we changed the order of the factors. To make things as simple as possible we always agree to use the canonical representation. The word “canonical” is just a fancy name for “natural,” and the most natural way to write a prime factorization is in increasing order of primes. So the canonical representation of 6 is 2 · 3. As another example, the canonical representation of 18 is 2 · 3 · 3. We can tidy this up a bit by rewriting 3 · 3 as 3². So the canonical representation of 18 is 2 · 3². If you are new to factoring, you may find it helpful to draw a factor tree. For example here is a factor tree for 18:

To draw this tree we started by writing 18 as the product 2 · 9. We put a box around 2 because 2 is prime, and does not need to be factored anymore. We then proceeded to factor 9 as 3 · 3. We put a box around each 3 because 3 is prime. We now see that we are done, and the prime factorization can be found by multiplying all of the boxed numbers together. Remember that we will usually want the canonical representation, so write the final product in increasing order of primes.

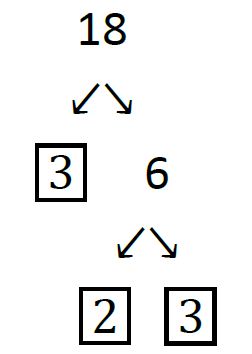
By the Fundamental Theorem of Arithmetic above it does not matter how we factor the number – we will always get the same canonical form. For example, here is a different factor tree for 18:

For practice, why don’t you try to draw a factor tree for 6137? Note that this is much more challenging than any number you will have to factor on the SAT. I’ll have the solution for you next week, so we can compare notes then. Remember, I am not looking for artistic merit – just make your factors clear in your drawing.

How many prime numbers are there?

This is going off on a bit of a tangent, but there are an infinite number of prime numbers. This was first proved by the ancient Greek mathematician Euclid.

Interestingly, the largest prime number found so far is  2^57,885,161 – 1. That’s a number with 17 million digits! I dare you to find the next one greater than that.

In the meantime, if you want to learn mathematical strategies to efficiently answer SAT math questions, I would suggest that you take a look at my 28 SAT Math Lessons Series. Click on the picture below for more information about these books.

If you liked this article, please share it with your Facebook friends:

And if you have any questions on this topic, then please post them on my Facebook wall:

I will answer your questions right away.

Speak to you soon!

Differences of Large Sums

The College Board sometimes likes to put seemingly difficult problems on the SAT where you are asked to find two large sums and then subtract them.

These problems always remind me of a little story about a young boy named Karl Gauss. The story actually directly relates to the strategy I will be discussing, so it’s worth taking this little detour.

The Boy Karl Gauss

In a math class, a teacher set her students to a task to keep them busy for a few minutes.

In an attempt to keep the class quiet, the teacher asked the students to take out a piece of paper and add up the integers from 1 to 100 by hand.

The teacher was hoping for the students to painstakingly write down 1, 2, 3, 4, 5 etc… to 100, and then add the numbers together to get an answer. The teacher knew that this problem would take some time to complete as she, herself, took several minutes to get the answer.

However, one of her students, Karl, handed the teacher a piece of paper with the correct answer almost immediately. In the top left corner of the sheet, he had neatly written 5,050.

Nothing else.

Surprised that the student had the correct answer so quickly, the teacher asked how he did it.

“Oh, this was really easy” said Karl, “1 + 100 = 101, 2 + 99 = 101, 3 + 98 = 101, 4 + 97 = 101… and so on. I could see that there are 100 pairs of numbers that add up to 101. So I multiplied 101 by 100 to get 10,100. I then divided by 2 because I added each number twice. So I wrote down the answer 5050.”

Let’s take one last look at Karl’s computation in an easy to read format as we will be using this format to solve SAT problems in just a moment.

    1   +   2   +  3   + … + 100
100 + 99  + 98  + … +  1
101 + 101 + 101 + … + 101

101 + 101 + 101 +…+ 101 = 100(101) = 10,100

10,100/2 = 5050

We are going to simulate Karl Gauss’s method above to get the answer to some seemingly difficult SAT math questions quickly and without making careless computational errors. Don’t worry – I will explain everything step by step.

This method is best understood with examples So let’s jump right in with SAT problems.

Example 1:

1. If x denotes the sum of the integers from 10 to 70 inclusive, and y denotes the sum of the integers from 80 to 140 inclusive, what is the value of y – x?

First, we write out each sum formally and line them up with y above x.

  80 + 81 + 82 + … + 140
10 + 11 + 12 + … + 70

Next subtract term by term.

  80 + 81 + 82 + … + 140
10 + 11 + 12 + … + 70
70 + 70 + 70 + … + 70

Now notice that we’re adding 70 to itself 70 – 10 + 1 = 61 times (by the fence-post formula – see last week’s blog post). This is the same as multiplying 70 by 61. So we get (70)(61) = 4270.

Example 2:

2. Let x ♦ y be defined as the sum of all integers between x and y. For example, 1 ♦ 4 = 2 + 3 = 5. What is the value of (60 ♦ 900) – (63 ♦ 898)?

As above, we write out each sum formally, line them up so that the numbers match up, and then subtract term by term.

     61 + 62 + 63 + 64 + … + 897 + 898 + 899
          64 + … + 897 
61 + 62 + 63 + 0 + …  … + 0 + 898 + 899

So the answer is 61 + 62 + + 63 + 898 + 899 = 1983.

Note that in each of the above problems, the numbers were lined up in different ways. It is usually pretty clear how to line up the numbers properly.

If you liked this article, please share it with your Facebook friends:

And if you have any questions on this topic, then please post them on my Facebook wall:

Speak to you soon!

Fence-posts in Advanced SAT Math Problems

It’s been a few weeks since I have provided you with some mathematical content, as I’ve been spreading the word on the release of my new book: The SAT Prep Official Study Guide Math Companion. Make sure you click on the below picture if you need detailed solutions to the problems from the ten practice tests in the College Board’s Blue Book.

Today I want to get a little more specific than usual and discuss a math concept that can be used to solve certain types of problems that appear every now and then on the SAT. Tutors tend not to spend much time (if any) helping students prepare for this type of problem as it seldom appears. But if you want an 800, then you want to be able to recognize all problem types and be able to answer them correctly (and quickly!).

This problem type involves what I like to call “Fence-Posting,” a technique that allows us to count the number of integers in a consecutive list.

The Fence-post Formula

The number of integers from a to b, inclusive, is b – a + 1.

The word inclusive means that we include the extreme values. For example, if we want to count the integers from 1 to 3, inclusive, we can easily see that there are 3 of them. Note that we do include the extreme values 1 and 3. Using the fence-post formula we get 3 – 1 + 1 = 3.

The most common error when attempting to count the integers in a list is to simply subtract the smallest value from the largest value (without adding 1). Note that in the previous example, this would give an incorrect answer of 2.

As another simple example, let’s count the number of integers from 5 to 12, inclusive, in two ways – directly and by fence-posting. First directly – the integers from 5 to 12 are 5, 6, 7, 8, 9, 10, 11, 12, and we see that there are 8 of them. Now using the fence-post formula we have 12 – 5 + 1 = 8. Note that the computation 12 – 5 gives an incorrect answer of 7.

If you ever happen to forget this little formula test it out on a small list of numbers as I just did in the two examples above. But it’s nice to have this one committed to memory so that it is there for you when you need it.

Let’s take a look at a couple of examples where we can use fence-posting to obtain an answer efficiently.

Example 1:

1. Set X contains only the integers 0 through 180 inclusive. If a number is selected at random from X, what is the probability that the number selected will be greater than the median of the numbers in X?

So first of all we need to find out how many integers there are in the set X. By the fence-post formula there are 180 – 0 + 1 = 181 integers in this set.

The median of the numbers in set X is 90 (note that in a set of consecutive integers the median is equal to the average of the first and last integer). Again, by the fence-post formula, we see that there are 180 – 91 + 1 = 90 integers greater than the median.

From this we see that the desired probability is 90/181 ~ 0.4972375691.

So we grid in .497. (Note that this is a grid-in question because I have not provided answer choices).

Remember with grid in problems, we have only four slots available. We can simply truncate the final answer that we get in our calculator to fit in the four slots.

Example 2:

2. How many numbers between 72 and 356 can be expressed as 5x + 3, where x is an integer?

This problem is more complicated than the first example, but we can use fence-posting to solve this one too: Let’s start by guessing x-values until we find the smallest and largest values of x satisfying 72 < 5x + 3 < 356. Since we have 5(13) + 3 = 68 and also 5(14) + 3 = 73 we see that 14 is the smallest value of x satisfying the inequality. Since 5(70) + 3 = 353 and 5(71) + 3 = 358, the largest value of x satisfying the inequality is 70. It follows by fence-posting that the answer is 70 – 14 + 1 = 57.

Note that we also used the strategy of taking a guess to solve this problem. I discussed this strategy in a previous blog post. You can find that information by clicking on the link.

Algebraic Solution:

Although I am not a fan of students using algebra on the day of the SAT, it is true that we could have also found the two extreme x-values algebraically:

72 < 5x + 3 < 356
69 < 5x < 353
69/5 < x < 353/5
13.8 < x < 70.6
14 ≤ x ≤ 70

We get the last inequality because x must be an integer. Notice that this last inequality is not strict. For those of you not familiar with this piece of math lingo, an inequality that is not strict means that it is ‘less than or equal to’ rather than just ‘less than’.

Next week, I will be showing a quick method for computing differences of large sums. You will also see how the fence-post formula can sometimes be useful in solving these types of problems. Click the following link to read this article: Differences of Large Sums

If you liked this article, please share it with your Facebook friends:

And if you have any questions on this topic, then please post them on my Facebook wall:

I hope all is well with you today.

I was with a friend of mine who was telling me a story about her son in his junior year of high school who is SAT obsessed.

This student has every problem in every available big brand name SAT prep book memorized. He can recite paragraphs of text relating to SAT concepts that are discussed in these prep guides.

However, when this student took the SAT and got his results he was shocked. And not shocked in a good way!

You see, even though this student studied and put in time (probably too much time in my opinion) he never studied by practicing problems that are exactly like those on the actual SAT. He was ‘unfamiliar’ with the  look/style of the problems. 

I, of course, recommended that my friend’s son practice problems found within the The Official Guide.

No matter who is helping students with their SAT prep – tutors, teachers, or even other students – we all agree that the practice tests in The Official Study Guide should be used during their preparation.

The reason for this is simple. The Official Guide, also known as the Blue Book, is the only SAT prep product written by The College Board, the same organization that creates the SAT. The 10 tests in the Blue Book contain problems similar to those that you can expect to see on test day.

Any student that is not practicing problems from the Blue Book is putting themselves at a serious disadvantage.

However, you may have noticed the distinct lack of detailed solutions with The College Board’s problems in the tests.

Although the problems in the blue book are fantastic, the solutions provided by the College Board are not so fantastic. In the actual Blue Book there are no solutions at all, and if you do have access to the College Board’s solutions then you have only a single solution (often involving time sapping complicated algebra) that may not be the best way to solve to the problem.

Before my students commence tutoring sessions with me they always complain that The Official Guide has no solution explanations.

This is why I have created The Complete Official SAT Study Guide Companion.

This book contains explanations for all the math problems in the tests in the Blue Book. My solutions are detailed, and often I show how problems can be solved in several different ways so that you can choose the methods that suit you the most on test day. 

Remember, unlike The College Board, I am not a fan of using tedious, time-consuming and complicated algebra to solve a question. My methods are quick, efficient and (dare I say!) fun. I use mathematical tricks, tips and strategies to solve problems and I detail my methods in a step by step way.

I have been tutoring SAT math for over 13 years now, and none of my students use complex methods to solve problems on test day. My students would much rather use the quickest and simplest method to solve a problem and then use the time they have left over to “recheck” their answers using a different method to make sure that they have not made a careless error.

My students are always happy with the score they get in SAT math!

You should certainly be using College Board practice tests to make sure that you are implementing strategies correctly and using your time wisely under pressure. You can easily use this guide to provide explanations to any problems you get incorrect during your practice. By reattempting the problems you get wrong with my methods, you will see how you have internalized more efficient ways to solve problems correctly.

This means you can expect a higher score on results day!

Problems to all 10 tests are here in this guide. You also get access to my solutions to the problems in the Official DVD Test.

All in all, I have provided you with the most effective and efficient solutions to every problem in 11 tests.

Click on this link to take you to the product page – you can buy an electronic or paperback version of the book.

Feel free to ask me any questions you may have about this new book.

All the best!

Hi all.

You may be aware that I have a YouTube channel featuring many of my SAT Math videos offering advice, tips and strategies as well as other test prep related material.

I would love for you to take a look at the channel and even subscribe to it so that you can be updated whenever I upload new content.

Below are all the videos I have made and uploaded to the channel so far. I am showing you these here in case you have not seen them before. A lot of the content in these videos is aimed at students who want advice to achieve an 800 score.

This first video details preparing for the SAT math in the correct way. This is actually my most popular video with the most views. It seems to me that YouTube viewers must like the content in this video. I hope you find this (and all the others) useful.

 

This second video shows the important SAT math formulas you need to remember to get a perfect or near perfect score in SAT math.

 

Next I have this video on how to make sure that you get those last few math questions on any math section of the SAT correct.

 

The video below is one of my first videos showing an SAT math strategy. The styling is different in this video compared to the others, but the content is just as good!

 

Here I am completing an SAT math section. I have done this to show how easy it is to finish the test with plenty of time left over to check your answers.

 

And here I am completing another SAT math section.

 

And here is the final SAT math test section being completed.

 

Next, I have a video providing advice on how best to take the SAT for an 800 in math.

 

This video is all about my latest series of books 28 SAT Math Lessons to Improve Your Score in One Month. These books are rather unique and so I felt as if I should explain what these SAT math prep books are and who they are for.

 

Whenever you are taking expert advice from someone you should always make sure that they are qualified to be giving such advice. This next video describes my SAT math qualifications and credentials.

 

Students often incorrectly use their first instinct to answer SAT problems on test day. Here I explain that using your gut instinct can be a dangerous method to answer SAT math problems – ultimately lowering your SAT math score.

 

Finally, here is video giving the pros and cons of paying for a professional SAT math prep course.

 

I hope you enjoy these videos and find them useful. I would really appreciate if you would ‘like’ each of the videos by pressing the thumbs up button, share them, and make comments. Of course, please subscribe to the channel as well.

Until next week!

Two weeks ago I mentioned that I wrote and released my detailed solutions to Test 8 in the College Board’s Official Guide (also known as the Blue Book). I released Test 8 first because my brand new laptop crashed just as I was completing Test 7. I’m still hurting from the loss, and do not want to re-write Test 7 just yet!  See last week’s post for details if you didn’t read it.

Today I would like to announce that I have completed the solutions to Test 9 in the College Board’s Blue Book. The Official SAT Study Guide Companion – Test 9 is now available.

As usual, this guide  often gives several different ways to solve a given problem. I always say that being able to solve any specific SAT math problem is not that important. After all, you will never see that specific problem on an SAT again. It is more important to learn as many techniques as possible that can be applied to a wide range of problems. This is what you will get in my solution guides.

The link to purchase this book is here. Solutions to tests 2,3 ,4, 5, 6 and 8 can also be found through the same link. These are available for $3 each. The solutions to the College Board’s Blue Book – Test 1 can be downloaded for free by subscribing to the 800 Initiation – a free online SAT math e-mail course that I know will boost your SAT scores on test day provided you internalize the advice and review the problems within it.