500 New SAT Math Problems
Just 19.99 on Amazon

Hi everyone! The latest edition of 500 New SAT Math Problems is now available in paperback from Amazon. This edition just has been modified from the previous edition to account for the changes on the Digital SAT.

The paperback is now on sale on Amazon for only $19.99. Note that once the sale ends (by the end of today), the price of this book will go up to $42.99. 

The promotion has ended. Thanks to everyone who participated. The book is now available at its regular price here: 500 New SAT Math Problems

If you have any questions, feel free to contact me at steve@SATPrepGet800.com 

Thank you all for your continued support!

A Trick For Free Two Day Shipping

I would like to finish this post with a little trick you can use to get free 2 day shipping on any of the books you decide to purchase without making any additional purchases. If you have never used Amazon Prime you can sign up for a free month using the following link.

Sign Up For Amazon Prime For Free

If you have already had a free trial of Amazon Prime you can simply open up a new Amazon account to get a new free trial. It just takes a few minutes! You will need to use a different email address than the one you usually use.

This next part is very important! After you finish your transaction, go to your Account, select “Manage my prime membership,” and turn off the recurring billing. This way in a month’s time Amazon will not start charging you for the service.

After shutting off the recurring billing you will still continue to receive the benefit of free 2 day shipping for one month. This means that as long as you use this new Amazon account for your purchases you can do all of your shopping on Amazon for the next month without having to worry about placing minimum orders to get free shipping.

Just be aware that certain products from outside sellers do not always qualify for free shipping, so please always check over your bill carefully before you check out.

Well I hope you decide to take advantage of this very special offer, or at the very least I hope you will benefit from my Amazon “free 2 day shipping trick.” Here is the link one more time:

Sign Up For Amazon Prime For Free

If you think your friends might be interested in this special offer, please share it with them on Facebook:

Thank you all for your continued support!

Turn Wrong Answers Into Right Answers

Today I want to discuss a common mistake that students make which leads to bad preparation.  The type of mistake I am about describe is especially common during preparation for standardized tests, and in particular the SAT (also note that this information is just as relevant to the ACT).

Although many SAT problems can be very difficult to solve, it is usually not very hard to understand the solutions. After getting a problem wrong, a good student will go over the solution until they understand it completely. Very often, however, the same student will misinterpret understanding the solution as being able to reproduce the solution on their own.

If after a student gets a question wrong I explain the correct way to do it and they understand my explanation, then more than likely if I ask them to solve the question 5 minutes later they will be able to do so. But it is important to realize that this does not mean that they will be able to solve a similar question (or even the exact same question) two months later during their SAT.

Understanding a solution and being able to reproduce it using short term memory does not necessarily lead to long term retention.

So what is the answer? How does a student make sure that they do not make the same mistake on their actual exam that they had made during their preparation?

The answer is quite simple.

During preparation, anytime you get a question wrong mark it off. You should have a special mark that is easy to see and recognize. Students of mine use stars, spirals or even sad faces.

Yes, the marks above were created in MS Paint! But I’m sure, you get the idea…

It is very important to mark off every single question you have gotten wrong regardless of the reason. Even if the only reason you got it wrong is because you accidentally hit a wrong button in your calculator or even if you got it wrong because you just misread one of the numbers in the problem – mark it off anyway! It is very easy to say “oh that was just a silly mistake – I know how to do this,” and then to never look at that problem again. This is a huge mistake. Let me repeat this one more time. Mark off EVERY question you get wrong. Every question! Not just the ones you do not understand. Every single one!

You must reattempt each question you get wrong at least four days later. Do not reattempt the problem the next day, or the day after that. If you only wait one or two days you may be getting the problem correct for the wrong reason. The question is too fresh in your mind. You need time to forget how you solved it. This way you can be sure that you understand how to solve the problem, as opposed to simply recalling the solution.

If you get the problem correct you can “unmark” it and remove it from the list of problems you need to redo. If you get it wrong again, then leave it marked off and reattempt it again at least four days later. You need to keep reattempting each problem you get wrong with at least four days between attempts. You should only stop reattempting a problem once you get it completely correct on your own without recalling exactly how the problem was solved. Only then can you know for certain that you have internalized the technique necessary to solve that problem.

If you follow the advice I have given here, then every time you get a problem right that you had previously gotten wrong, you will be one step closer to a perfect score.

I want you to treat this message in the same way as I recommend you treat a question you answer incorrectly. Come back to this in 4 days time. Re-read and internalize. I am certain you will benefit from the information here if you make it a part of your long term memory..

And if if you would like lots of practice problems I suggest you take a look at my 28 SAT Math Lessons Series. Click on the picture below for more information about these books.

If you liked this article, please share it with your Facebook friends:

And if you have any questions on this topic, then please post them on my Facebook wall:

The title of this post may seem a bit strange, but I do have an explanation for you… In the future I may look back on the following story and laugh, but right now that’s not the case.

I have been releasing my detailed solutions to the tests in the College Board’s Official SAT Study Guide in numerical order. I planned to write Test 1 first and Test 10 last.

My master plan was going well. I released the solutions to SAT 1 through 6 and I had just completed Test 7.

But then my laptop crashed. Not just crashed as in “froze” or “displayed the blue screen of death” – my laptop’s innards were utterly destroyed; cut down in the prime of its life – it was only a month old!

Anyway, upon restarting my computer, my data was gone. All wiped away. Nothing left. All my previous work disappeared. My so-close-to-completion solution guide for Test 7 was gone. Not only did I lose the work I put into my Get 800 SAT math books, but I also lost a month’s worth of research related to my work at Hofstra University.

The ironic thing is that I just started backing up my data when this happened!

I took my laptop to an expert to see if the data could be recovered, but there was no hope. Accepting that you have lost all your data is a a tough pill to swallow. But I quickly moved on to creating my solutions to Test 8. I do not want to rewrite my solutions to Test 7 just yet, as I am still mourning the loss of the work I had put in to creating it.

So today I would like to announce that I have completed the solutions to Test 8 in the College Board’s Blue Book. “The Official SAT Study Guide Companion – Test 8” is now available.

This guide that I have written often has several different ways to solve a given problem. I always say that being able to solve any specific SAT math problem is not that important. After all, you will never see that specific problem on an SAT again. It is more important to learn as many techniques as possible that apply to a wide range of problems. This is what you will get in my solution guides.

Here is the link to purchase the solutions to Test 8 of the College Board’s Blue Book. Solutions to tests 2, 3, 4, 5 and 6 can also be found through the same link. These are all available for $3 each. The solutions to Test 1 can be downloaded for free by subscribing to the 800 Initiation – a free online SAT math e-mail course that I know will boost your SAT scores on test day provided you internalize the advice and review the problems within it.

Changing Averages To Sums

A problem involving averages often becomes much easier when we first convert the averages to sums. We can easily change an average to a sum using the following simple formula.

Sum = Average · Number

Many problems with averages involve one or more conversions to sums, followed by a subtraction.

Examples:

Try to answer the following question using this strategy. Do not check the solution until you have attempted this question yourself.

1. The average (arithmetic mean) of three numbers is 100. If two of the numbers are 80 and 130, what is the third number?

               (A)   70
               (B)   80
               (C)   90
               (D)   100
               (E)   110

In this case we are averaging 3 numbers. So the Number is 3. The Average is given to be 100. Thus, the Sum of the 3 numbers is 10 · 3 = 300. Since two of the numbers are 80 and 130, the third number is 300 – 80 – 130 = 90, choice (C).

Got it? Well here is another, slightly more advanced, problem where this technique is useful:

2. The average of x, y, z  and w is 15 and the average of z and w is 11. What is the average of x and y?

The Sum of x, y, z and w is 15 · 4 = 60. The Sum of z and w is 11 · 2 = 22. Thus, the Sum of x and y is 60 – 22 = 38. Finally the Average of x and y is 38/2 = 19.

Notice how we actually used the formula “Sum = Average · Number” twice here. For those of you that require further clarification, here are the calculations in more detail.

If you liked this article, please share it with your Facebook friends:

Changing Averages To Sums

More On Remainders

Welcome to the third and final part of this thread on remainder problems. In the first part we discussed some calculator algorithms for finding remainders. You can review that article here: SAT Remainder Problems – Part 1

Last week we discussed the cyclical nature of remainders. You can review that article here: SAT Remainder Problems – Part 2

I decided to spend three blog posts on this subject because many of my students in a mid-level scoring range seem to have a difficult time with SAT remainder questions.

Solution To Last Week’s Remainder Problem

So where were we? I left you with an SAT math problem to solve using the information I provided regarding the cyclical nature of remainders. Let’s find out how you did.

The problem again:

What is the least positive integer greater than 4 that leaves a remainder of 4 when divided by both 6 and 8?

We first find the least positive integer greater than 4 that is divisible by both 6 and 8. This is the least common multiple of 6 and 8 which is 24.

We now simply add the remainder.

24 + 4 = 28.

Thus, the answer is 28.

If you don’t know how to find the least common multiple of two numbers, it’s okay. You can just let your TI-84 calculator do it for you. In your calculator press MATH, scroll right to NUM, and press 8 for lcm. Then type 6,8) and you will get an output of 24. So lcm(6,8)=24.

For more information on the lcm, see the following article: Greatest Common Divisor (GCD) And Least Common Multiple (LCM)

Remainders In Disguise

The College Board loves to put their remainder problems in disguise. In fact, most remainder problems on the SAT do not even have the word “remainder” in the question! So how can you be expected to know when to find a remainder? The answer is quite simple. If the problem mentions some kind of sequence that keeps repeating over and over, then the problem may be asking you to find a remainder.

Remember this and you will recognize a remainder problem every time. In fact, this is such a key point I am going to repeat this in bold for you!

If the problem mentions some kind of sequence that keeps repeating over and over, then the problem may be asking you to find a remainder.

Let’s take a look at a standard example of such a question:

Cards numbered from 1 through 2013 are distributed, one at a time, into nine stacks. The card numbered 1 is placed on stack 1, card number 2 on stack 2, card number 3 on stack 3, and so on until each stack has one card. If this pattern is repeated, each time beginning with stack one, on which stack will the card numbered 2013 be placed?

So this is the end of this three part thread on remainders. If you liked this article, please share it with your Facebook friends:

And if you have any questions on this topic, then please post them on my Facebook wall:

See you soon!

Remainder Problems

Last week I introduced the idea of solving remainder problems without using long division. We may as well take advantage of the fact that we can use our T-84 calculator on the SAT to solve seemingly difficult SAT math questions with ease. If you haven’t done so already, please read part 1 before continuing with this article: SAT Remainder Problems – Part 1

Solution To Last Week’s Problem

In the last post I left you with a cliffhanger of a problem to solve with your calculator. Below is the solution. Let’s see if you got the problem correct and which method you used to solve the problem.

Here is the question again:

What is the remainder when 15,216 is divided by 73?

If you recall I gave three methods to solve such a problem, the first of which is by using long division. Since this post is about solving problems without using long division, let’s skip that method and jump straight to the much quicker and more efficient calculator algorithms – great for use on test day!

Method 2 – First Calculator Algorithm:

Step 1: Perform the division in your calculator:

15,216/73 ~ 208.438

Step 2: Multiply the integer part of this answer by the divisor:

73*208 = 15,184

Step 3: Subtract this result from the dividend to get the remainder:

15,216 – 15,184 = 32.

And here is an alternative:

Method 3 – Second Calculator Algorithm:

Step 1: Perform the division in your calculator:

15,216/73 ~ 208.438

Step 2: Subtract off the integer part of this result:

ANS – 208 ~ .438

Step 3: Multiply this result by the divisor:

73*ANS = 32.

Did you get this problem correct? Which method did you use? Try using both methods and then choose the one that you will be most comfortable with on the day of the SAT. And remember: the best way to get comfortable with a new technique is to practice with it!

When we divide 3 by 3 we get a quotient of 1 and a remainder of 0. In other words,

3 = 3(1) + 0.

When we divide 4 by 3 we get a quotient of 1 and a remainder of 1. In other words,

4 = 3(1) + 1.

Now we have to be careful. When we divide 6 by 3, the quotient is now 2 and the remainder is 0. That is, 6 = 3(2) + 0.

Now let’s see if you can apply this information to an actual SAT math problem – the type you can expect to see in the College Board’s Blue Book. Here is a nice medium difficulty problem I like to give to my own SAT math students:

What is the least positive integer greater than 4 that leaves a remainder of 4 when divided by both 6 and 8?

Do you know how to go about solving this? You can find the answer in part 3 of this article here: SAT Remainder Problems – Part 3

You may want to check out my 28 SAT Math Lessons Series for more SAT math remainder problems. Click on the picture below for more information about these books.

If you liked this article, please share it with your Facebook friends:

And if you have any questions on this topic, then please post them on my Facebook wall:

And now read part 3 of this article here: SAT Remainder Problems – Part 3

I’m sitting here with a Japanese friend of mine who is a math teacher and also has a nice website offering free math help to Japanese high school students.

I’m looking at the kind of math that an equivalent 10^th grade student would have to study (1st grade in Japan) – and it’s mind blowing. It’s the kind of material that an SAT Mathematics Level 2 Subject Test student would have to master to get a top score in the exam.

I just wanted to share his website with you: 13^th Note. 

You probably will not be able to read much of it, as it is in Japanese. But I did want to spread the word for the good work that he is doing – in Japan, the math books that one has to learn from are set by the Government and are even more dull than the College Board’s books. His free books are becoming very, very popular in Japan as they are easy to understand and very concise.

I feel that the work we are doing is very similar, albeit in different countries.

Perhaps if you are a Japanese student in America, you can take a look at this site and see how different the subjects are.

Keep up the good work, Kutomi!

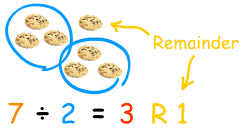SAT Remainder Problems

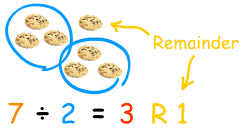
For the next few weeks I would like to help you to understand SAT math problems involving remainders. This will be the first of three such articles.

SAT math problems with remainders seem to give students a difficult time and limit SAT scores. This is mostly because a fundamental step in solving the problem is often missed – performing long division.

You cannot solve a remainder problem by simply dividing in your calculator. There are, however, calculator algorithms that can give you the answer very quickly. We will talk about these a bit later in this post.

A common error that students make is to perform a division calculation on their TI-84 calculator or similar device, and simply take the first number after the decimal point and use this digit as the answer to the problem. This will usually result in a wrong answer.

Example:

Suppose we are asked “Find the remainder when 14 is divided by 4.”

In your calculator, 14/4 = 3.5.

Penciling the number 5 as your answer would be incorrect – seriously incorrect. This “5” is actually part of the answer to the question “What is 14 divided by 4?” But it has nothing to do with the remainder.

Students that are currently scoring in the mid-range in PSAT/SAT math seem to have the biggest problem with remainder questions. Many cannot perform long division correctly, and some do not even realize that long division is needed. This keeps many students from getting their SAT scores to the next level.

Let’s take a look at how we can solve the problem of finding the remainder when 14 is divided by 4 in three different ways. This problem may seem very basic for some of you, but let’s go over it anyway to make sure we have a strong foundation before solving more difficult remainder problems.

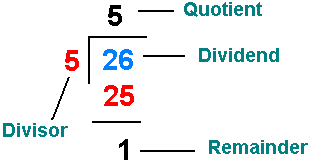
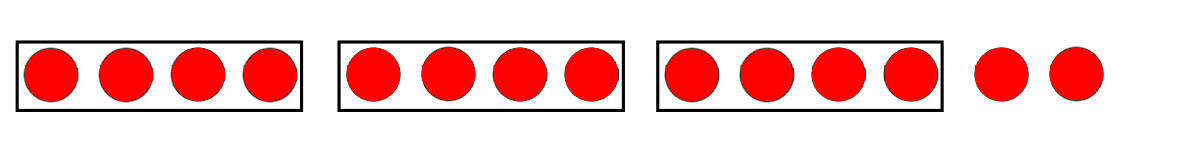
Method 1 – Long Division: So 4 goes into 14 three times with 2 left over. In other words, 14 = 4(3) + 2. So we see that the remainder when we divide 14 by 4 is 2.

Let’s break this down step by step:

Count the number of groups of 4 objects that can be formed from 14 objects. Place this number over the 4 that is inside the division symbol.

Multiply this resulting  number 3 by the divisor 4. Place this number below the 4 that is inside the division symbol.

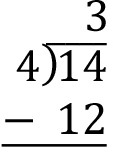
Subtract the resulting number 12 from the dividend 14.

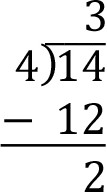
The quotient 3 appears above the division symbol and the remainder 2 appears at the bottom.

Below is a visual representation of 14 divided by 4. In the figure below we are grouping 14 objects 4 at a time. Note that we wind up with 3 groups (the quotient) and 2 extra objects (the remainder).

Method 2 – First Calculator Algorithm:

Step 1: Perform the division in your calculator: 14/4 = 3.5

Step 2: Multiply the integer part of this answer by the divisor: 4*3 = 12

Step 3: Subtract the above result from the dividend to get the remainder:

14 – 12 = 2.

Method 3 – Second Calculator Algorithm:

Step 1: Perform the division in your calculator: 14/4 = 3.5

Step 2: Subtract off the integer part of this result: ANS – 3 = .5

Step 3: Multiply this result by the divisor: 4*ANS = 2.

Note that these calculator algorithms work exactly the same no matter how large the numbers are that you are dividing.

Exercise:

As an additional exercise you should try to find the remainder when 15,216 is divided by 73 by using one of these calculator algorithms. Figure out which of the two algorithms you prefer. You can find solutions here: SAT Remainder Problems – Part 2

More information on remainders can be found in my 28 SAT Math Lessons Series. Click on the picture below for more information about these books.

If you liked this article, please share it with your Facebook friends:

And if you have any questions on this topic, then please post them on my Facebook wall:

For part two of this article please click the following link: SAT Remainder Problems – Part 2

Those of you that have been following this blog will have noticed that I have been furiously releasing new SAT math products that will help you to increase your score on test day and to improve your overall application to get into college.

Today I would like to announce that I have completed the solutions to Test 6 in the College Board’s Blue Book. “The Official SAT Study Guide Companion – Test 6” is now available.

Maybe you are wondering why I am working so hard to create these solutions. Well, in the blue book there are no solutions provided at all, and if you do have access to the College Board’s solutions then you have only a single solution which, in my opinion, may not always be the best way to solve that problem. The College Board’s solution is often a very ‘mechanical’ way to solve the problem without the use of any of my  efficient and effective strategies (i.e. mathematical hints, tips and tricks).

This guide that I have written often has several different ways to solve a given problem. I always say that being able to solve any specific SAT math problem is not that important. After all, you will never see that specific problem on an SAT again. It is more important to learn as many techniques as possible that apply to a wide range of problems. This is what you will get in my solution guides.

The link to purchase this book is here. Solutions to tests 2,3 ,4, and 5 can also be found through the same link. These are all available for $3 each. The solutions to the College Board’s Blue Book – Test 1 can be downloaded for free by subscribing to the 800 Initiation – a free online SAT math e-mail course that I know will boost your SAT scores on test day provided you internalize the advice and review the problems within it.

Helping Others

This week I would like to share an approach to improving your own SAT math score by helping other students to improve their score. Every student I tutor is encouraged to pass along their knowledge to other students as there are many benefits for both students.

I believe that if you want to take yourself to the next level without too much effort, simply teach what you already know to someone else. Helping someone else to learn something new requires a much deeper understanding of the underlying material than simply having superficial knowledge of the subject matter. By engaging with someone else with less understanding than you, you will find that you often have to be creative with your explanations in order to get the other person to acquire a complete understanding of what you are trying to teach. This will force you to think about the subject matter in different ways. A nice side effect of this process is an overall improvement in both your current knowledge and your critical reasoning skills.

This is especially useful for SAT prep and also mathematics in general where it often helps to solve problems by thinking “outside the box.” By helping others to understand how to solve problems you will be forced to review the tips, tricks and techniques that you already know. This will help you to internalize them more quickly so that you will be able to more easily identify problems where a specific strategy will be useful, and you will become more adept at using them.

Ways To Help Others

There are several ways to help others:

1. You can find students in your school (or elsewhere) whose current score levels are below yours and offer to tutor them in a formal setting.
2. You can let other students know that whenever they are stuck on an SAT problem, they should see you, and you will be happy to do your best to provide a solution for them.
3. You can answer questions on forums where students post SAT questions. Feel free to answer questions in the Get 800 SAT/ACT Prep Forum, and post the questions that you are having trouble with yourself.

Benefits Of Helping Others

And now here are some of the benefits of helping others with their SAT prep:

1. You will be forced to review SAT material that you have already learned.
2. You will be more likely to internalize strategies that you are familiar with so that they’re application becomes faster and more reliable.
3. You will begin developing a deeper understanding of the underlying mathematics leading to a higher level of mathematical maturity.
4. You will improve your problem solving and critical reasoning skills at a faster rate than by simply learning new material.
5. You will be providing a service to others by helping them improve their SAT scores.

So get out there and offer your tutoring services to someone who needs your help. You will be providing an invaluable service to another person. They will certainly be grateful for your help.

Of course, this applies not only to improving your SAT math score but to anything in life that you want to perform well in: be it college, sports, or even just playing video games!

And what about the fact that primarily you’re actually helping yourself to get into your college of choice? Well, we’ll keep that as our little secret.

This week I had a question posted up on my Facebook Timeline by a student in regards to being able to increase one’s math score by the next SAT in March.

In the same question the student states that most of his errors are just “careless” mistakes. I redirected him to my article on avoiding careless mistakes, but let me just repost our whole conversation here as it has information relevant to a lot of other students. I hope you find this useful…

Student: Hi Dr. Steve, I feel as if I have hit a plateau because I can’t really score higher than a 710. I always do practice sections from the College Board Blue Book and miss around 1-2 questions per section. The questions I get incorrect are usually medium questions and not hard. I believe I make careless mistakes… What should I do? I also tend to do A LOT of practice sections. Could this negatively affect my score? My exam is on March 9th and I would like to score 750+(preferably an 800). Is increasing from a 700 to 750+ in just weeks impractical?

Dr Steve: The increase you are looking for is definitely possible. It sounds like all of your prep right now consists of doing practice tests from the College Board’s Blue Book. You should stop this and start prepping in a more efficient way. Now I also need to know what you mean by the word “usually” when you say that you usually get medium questions incorrect. Does that mean on any given practice test you get more medium questions wrong, or that you practically never get hard questions wrong at all? This distinction is important. Let me start with a few suggestions to get you on the right path.

1. If you haven’t done so already, read my article on avoiding careless errors here.
   
2. Go back through all the practice tests you took and start posting up the questions you got wrong here on my Facebook wall. Also explain what the error you made was, no matter how silly or careless you think the errors were. We can then discuss several different ways to solve these problems and how to avoid these errors in the future.
3. Are you proficient in the really basic strategies such as plugging in answer choices, picking numbers, taking guesses, etc. If not, then you need to start practicing lots of problems using these strategies even if you know more efficient ways to solve them. The Intermediate Course from my “28 Lessons” series emphasizes these strategies in a systematic way. The Advanced Course reviews these strategies very quickly in the first lesson, but still gives solutions using these techniques throughout the book. In your case, if you did decide to get one of these, I would probably recommend the Advanced, but make sure you are practicing solving as many of these problems as you can using both basic and advanced strategies. If you choose to continue using only the materials you already have, then again, make sure you are practicing both basic and advanced techniques.
4. Let me emphasize that as you practice solving SAT math questions, try to solve each one in 2 to 4 ways. Possibly using a basic strategy, a more advanced strategy, the way you would do it in school, and/or the quickest way you can think of. Remember – you will never see any specific math problem that you do on your SAT – it’s more important to learn techniques than it is to solve a specific problem.
5. To really improve your score do every problem you get wrong about once per week until you get that question right on your own without making any “careless” errors. Make sure you wait at least several days before reattempting a problem you have gotten wrong. You don’t want to get it right because you recall the mistake you made. You want to get it right because you are no longer falling into the trap you previously fell for.

Student: Well I can confidently say that I know most, if not all, of your strategies (I bought your book and went through the level 5 questions of your book since I got all level 4 questions correct) and that I know all the math involved in most, if not all problems. Out of all the sections I have ever taken, I have only been totally clueless in only like 2 problems (I’ll try to find them; they are questions from the online Free practice test by the College Board). By “usually” I mean I practically never get a hard question wrong unless I make a silly mistake or get tricked, which is quite rare. Since I know not to follow my intuition on hard questions, I tend to check over my work like 2-3 times. On medium questions, however, I tend to work really fast through them. The reason I do this is because it can sometimes take me quite a while to solve some hard questions even if I’m using strategies. Another thing, the way I attack the SAT math section is by just going once through the whole section. Even if I feel that I got all the answers right, I somehow made a mistake in one without noticing. I have question: What is the maximum amount of questions I can miss and still get a 750 or higher? Or does that change from test to test?

Oh wow, I looked back to a problem that I was totally clueless on and solved it in less than 30 seconds.

Dr Steve: To guarantee a 750 you can only get 2 questions wrong (omitting them or getting them wrong is equivalent here since the .5 loss will be rounded up). However, the scaling at the high end is very inconsistent, so depending on the overall difficulty of the test you may be able to omit 1 or possibly even 2 more.

Based upon your last response, here are a few suggestions:

1. Have you watched the 3 videos of me completing the 3 math sections of an SAT from the College Board Blue Book in about 9 minutes each? I think it would be useful to just observe my process. Especially pay attention to how I move quickly but how I do not rush and I even look over my work pretty often before moving on. It is very important not to rush through easier questions. Don’t try to emulate what I’m doing – you need to develop speed at your own pace – just observe.
2. For at least the next week focus on one problem at a time (no full practice tests). Go back and pick out problems that have taken you more than 1 minute. Really take the time to work out the problem several different ways and try to really internalize these methods. Then pay special attention to the one that was quickest.
3. In about a week take another practice test and really focus on NOT RUSHING. Pace yourself correctly meaning if you are working on a problem for about 30 seconds and you’re still confused by it, mark it off and move on – come back to it later – don’t worry – you’ll get to it, and as long as you’re pacing yourself correctly you will have plenty of time to finish the section, go back and work on the ones you skipped, and you may even have several minutes to go back and redo many of the problems one more time. You may want to read this article.”

The student replied that he would look at the additional material.

I wish him and all other students taking the March 2013 SAT the best of luck in achieving an exceptional math score..